Study on Uncertainty Analysis Method of Fast Reactor Based on Covariance Matrix Sampling
-
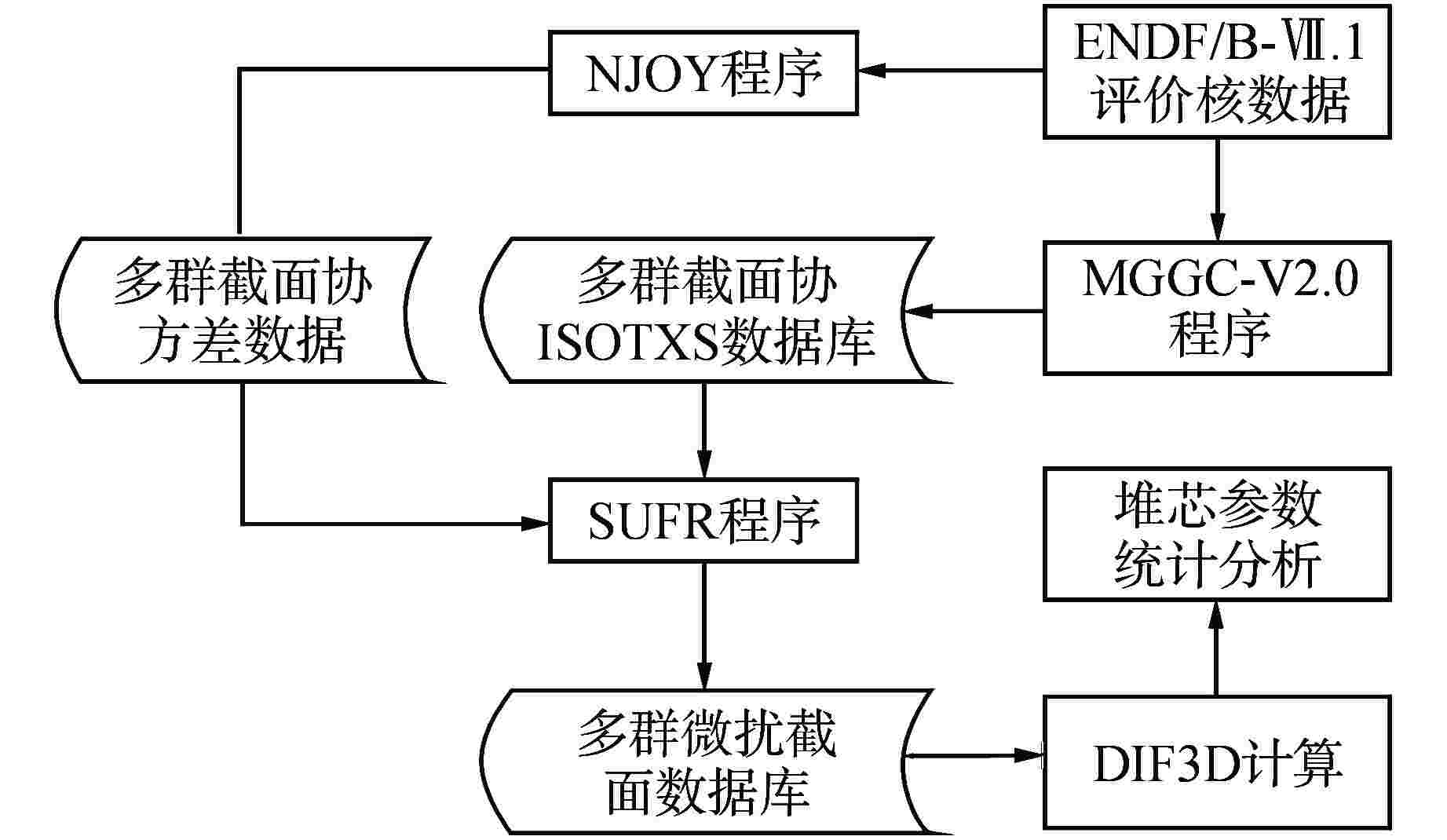
摘要: 基于传统统计学抽样的不确定性分析方法由于算法简单、程序容易实现及同时考虑高阶效应受到国内外广泛关注,但上述方法通常需要大量样本才能保证响应量计算精度。研究发现,产生以上现象的原因是抽样样本质量不高。通过改进抽样方法,面向协方差矩阵抽样时小样本量可以保证较高的计算精度。文中首先从理论上证明了面向协方差矩阵抽样方法的可行性,用简单测试题对其进行验证。在此基础上,使用自主开发的快能谱反应堆敏感性和不确定性分析程序SUFR,选取国际快堆基准装置ZPR-6/7,计算多个核素不同反应类型的核截面引起的有效增殖因子(keff)的不确定度,并与使用确定论方法计算的不确定度进行对比。结果表明,使用面向协方差矩阵抽样的情况下,样本量为50时,2种方法计算的不确定度偏差均低于1.3%。由此说明,面向协方差矩阵抽样方法可以很好地解决传统抽样方法计算不确定度时存在的问题,且SUFR程序面向协方差矩阵抽样功能的开发是正确的,该方法是对传统抽样方法的进一步发展。Abstract: The uncertainty analysis methods based on traditional statistical sampling have received widespread attention in China and other countries due to their simple algorithms, easy realization of codes, and consideration of high-order effects. However, these methods usually require a large number of samples to ensure the calculation accuracy of response variables. As found in the study, this phenomenon occurs because of the poor quality of the samples. After a covariance matrix sampling is used instead of the traditional sampling method, a small sample size can also ensure a high calculation accuracy. This paper firstly demonstrates theoretically the feasibility of the covariance matrix sampling method, and verifies it with simple tests. On this basis, this paper, using the self-developed fast spectrum reactor sensitivity and uncertainty analysis code - SUFR and the international reference configuration for fast reactor ZPR-6/7, calculates the uncertainty of effective multiplication factor (keff) caused by the nuclear cross sections of different reaction types of multiple nuclides, and compares the calculation results with the uncertainty calculated using the deterministic method. As demonstrated by the results, if the covariance matrix sampling is used, with a sample size of 50, the uncertainty deviation calculated in the two methods each is below 1.3%. This indicates that the use of the covariance matrix sampling method can solve the problems present in the use of the traditional sampling method to calculate uncertainty, and that it is appropriate to develop the SUFR code function against the covariance matrix sampling. This method represents a further development of the traditional sampling method.
-
Key words:
- Statistical sampling /
- Uncertainty analysis /
- Sodium-cooled fast reactor /
- SUFR
-
表 1 4种情况下的二维随机样本协方差矩阵
Table 1. Two-Dimensional Random Sample Covariance Matrix in Four Cases
样本量 方法1 方法2 方法3 方法4 50 $\left( {\begin{array}{*{20}{c}} {{\text{4}}{\text{.199}}}&{ - {\text{1}}{\text{.219}}} \\ { - {\text{1}}{\text{.219}}}&{1{\text{1}}{\text{.859}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.999}}}&{0.{\text{009}}} \\ {0.{\text{009}}}&{{\text{9}}{\text{.001}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.983}}}&{ - {\text{0}}{\text{.227}}} \\ { - {\text{0}}{\text{.227}}}&{{\text{8}}{\text{.831}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {\text{4}}&0 \\ 0&{\text{9}} \end{array}} \right)$ 200 $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.301}}}&{ - {\text{0}}{\text{.422}}} \\ { - {\text{0}}{\text{.422}}}&{{\text{9}}{\text{.259}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.995}}}&{{\text{0}}{\text{.050}}} \\ {0.{\text{050}}}&{{\text{9}}{\text{.011}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.965}}}&{ - {\text{0}}{\text{.211}}} \\ { - {\text{0}}{\text{.211}}}&{{\text{8}}{\text{.858}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {\text{4}}&0 \\ 0&{\text{9}} \end{array}} \right)$ 103 $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.863}}}&{ - {\text{0}}{\text{.082}}} \\ { - {\text{0}}{\text{.082}}}&{{\text{9}}{\text{.147}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{4}}{\text{.000}}}&{0.{\text{001}}} \\ {0.{\text{001}}}&{{\text{9}}{\text{.000}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.886}}}&{ - {\text{0}}{\text{.129}}} \\ { - {\text{0}}{\text{.129}}}&{{\text{8}}{\text{.758}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {\text{4}}&0 \\ 0&{\text{9}} \end{array}} \right)$ 104 $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.957}}}&{0.{\text{077}}} \\ {{\text{0}}{\text{.077}}}&{{\text{8}}{\text{.963}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{4}}{\text{.000}}}&{-{\text{ 0}}{\text{.003}}} \\ {-{\text{ 0}}{\text{.003}}}&{{\text{9}}{\text{.000}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {{\text{3}}{\text{.884}}}&{{\text{0}}{\text{.004}}} \\ {{\text{0}}{\text{.004}}}&{{\text{8}}{\text{.739}}} \end{array}} \right)$ $\left( {\begin{array}{*{20}{c}} {\text{4}}&0 \\ 0&{\text{9}} \end{array}} \right)$ 表 2 2种方法计算的多群截面引起的keff不确定度
Table 2. Uncertainty of keff due to Multigroup Cross Section Calculated by Two Methods
核素 反应类型 面向协方差矩阵抽样
方法/10−4确定论方法/10−4 相对偏差/% 235U σγ 2.3783 2.3783 0 σelas 0.01998 0.019980 0 σinel 0.069764 0.069779 −0.02 σf 0.50225 0.50224 0 v 0.79784 0.79784 0 238U σγ 33.491 33.408 0.25 σelas 9.8896 9.8097 0.81 σinel 64.832 65.496 −1.01 σf 4.0966 4.1066 −0.24 v 15.201 15.199 0 239Pu σγ 31.183 31.183 0 σelas 1.0731 1.0728 0.023 σinel 5.6450 5.7155 −1.23 σf 23.837 23.843 −0.02 v 7.1727 7.1727 0 56Fe σγ 10.373 10.352 0.21 σelas 9.4448 9.3937 0.54 σinel 10.954 10.922 0.29 23Na σγ 1.5406 1.5540 −0.86 σelas 4.1418 4.1332 0.21 σinel 7.0291 7.0317 −0.04 -
[1] SALVATORES M, JACQMIN R. International evaluation co-operation volume 26: Uncertainty and target accuracy assessment for innovative systems using recent covariance data evaluations: NEA/WPEC-26, ISBN 978-92-64-99053-1[R]. Paris: OECD Nuclear Energy Agency, 2008. [2] OECD. International evaluation co-operation volume 33: methods and issues for the combined use of integral experiments and covariance data: NEA/WPEC-33, NEA/NSC/WPEC/DOC(2013)445[R]. Paris: OECD, 2013. [3] RIMPAULT G, BUIRON L, STAUFF N E, et al. Objectives and status of the OECD/NEA sub-group on uncertainty analysis in modelling (UAM) for design, operation and safety analysis of SFRs (SFR-UAM)[C]//International Conference on Fast Reactors and Related Fuel Cycles: Next Generation Nuclear Systems for Sustainable Development (FR17). 2017 [4] PERFETTI C, REARDEN B. CE TSUNAMI-3D algorithm improvements in SCALE 6.2[J]. Transactions of the American Nuclear Society,2016, 114(6): 948. [5] KODELI I. The SUSD3D code for cross-section sensitivity and uncertainty analysis - recent development, invited[J]. Transactions of the American Nuclear Society, 2011, 104: 791-793. [6] WILLIAMS M L, ILAS G, JESSEE M A, et al. A statistical sampling method for uncertainty analysis with SCALE and XSUSA[J]. Nuclear Technology, 2013, 183(3): 515-526. doi: 10.13182/NT12-112 [7] 刘勇. 基于微扰理论的反应堆物理计算敏感性与不确定性分析方法及应用研究[D]. 西安: 西安交通大学, 2017. [8] 万承辉. 核反应堆物理计算敏感性和不确定性分析及其在程序确认中的应用研究[D]. 西安: 西安交通大学, 2018. [9] 胡泽华,王佳,孙伟力,等. 基准模型keff对核数据的灵敏度分析及不确定度量化[J]. 原子能科学技术,2013, 47(S1): 312-317. [10] 胡泽华,叶涛,刘雄国,等. 抽样法与灵敏度法keff不确定度量化[J]. 物理学报,2017, 66(1): 012801. doi: 10.7498/aps.66.012801 [11] SUI Z J, CAO L Z, WAN C H, et al. Covariance-oriented sample transformation: a new sampling method for reactor-physics uncertainty analysis[J]. Annals of Nuclear Energy, 2019, 134: 452-463. doi: 10.1016/j.anucene.2019.07.001 [12] 马续波,刘佳艺,徐佳意,等. 相关变量随机数序列产生方法[J]. 物理学报,2017, 66(16): 160201. doi: 10.7498/aps.66.160201 [13] SIMTH M A, LELL R M, MONEO P, et al. ZPR-6 ASSEMBLY 7: A cylindrical assembly with mixed (pu-u)-oxide fuel and sodium with a thick depleted-uranium reflector: NEA/NSC/DOC(95)03/VI[R]. Argonne National Laborary, 2003. [14] MACFARLANE R E, MUIR D W, BOICOURT R M, et al. The NJOY nuclear data processing system version 2012: LA-UR-12-27079[R]. Los Alamos: Los Alamos National Laboratory, 2012. [15] DERSTINE K L. DIF3D: A code to solve one-, two-, and three-dimensional finite-difference diffusion theory problems: ANL-SF-12-048[R]. Argonne: Argonne National Laboratory, 1984. -





 下载:
下载: