Distortion Evaluation of Natural Circulation Characteristic Curves Based on Scaled-down Methodology
-
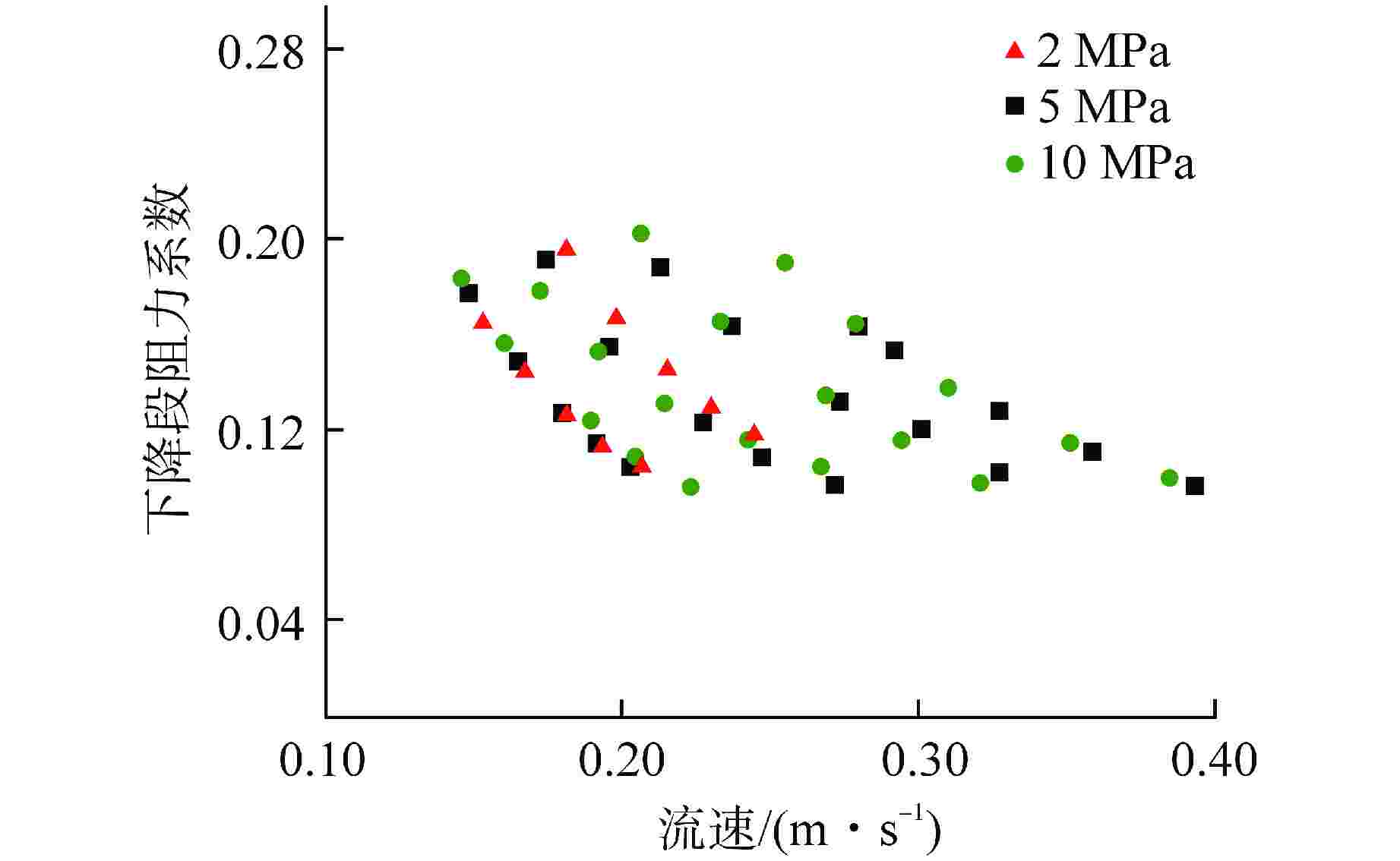
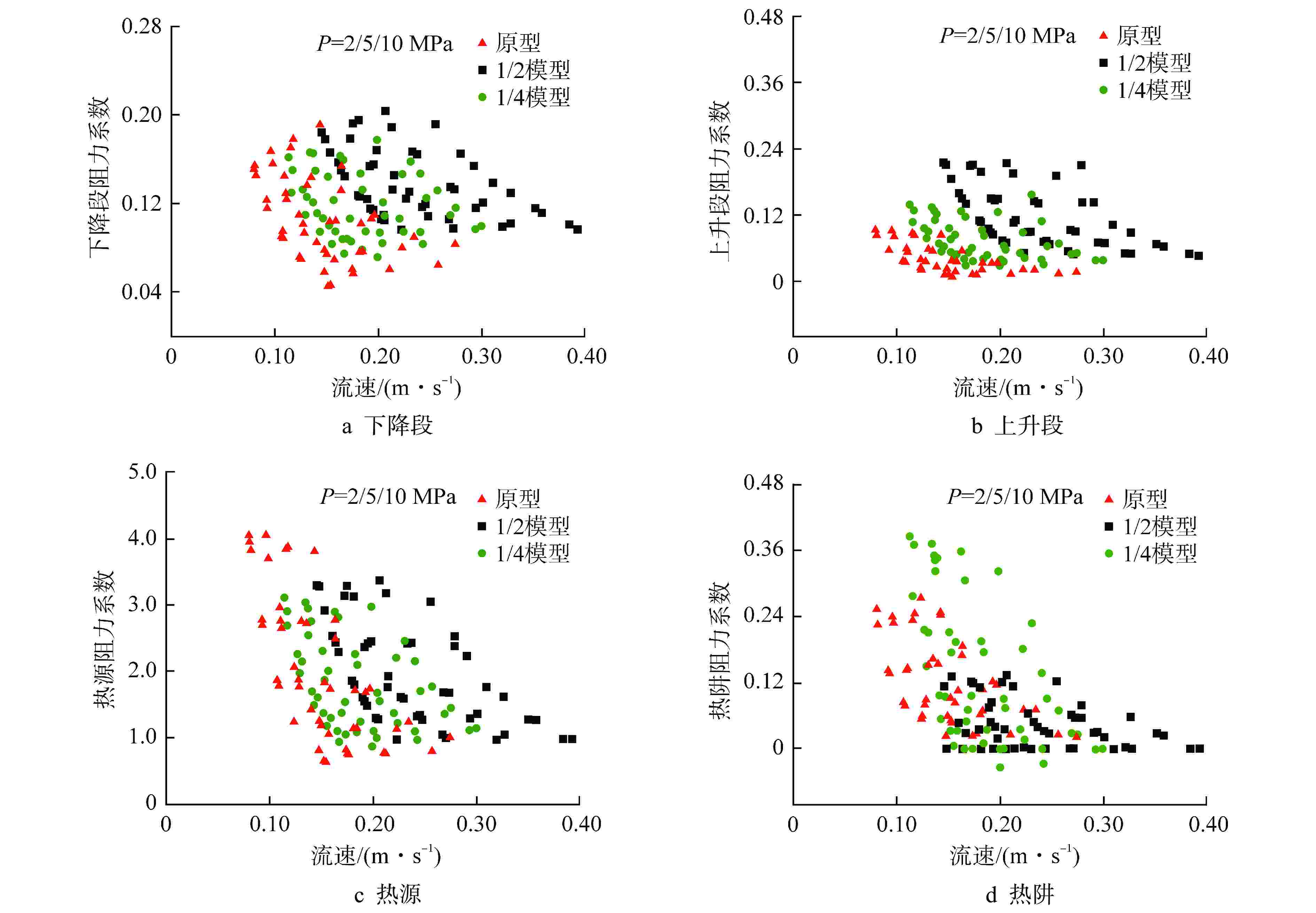
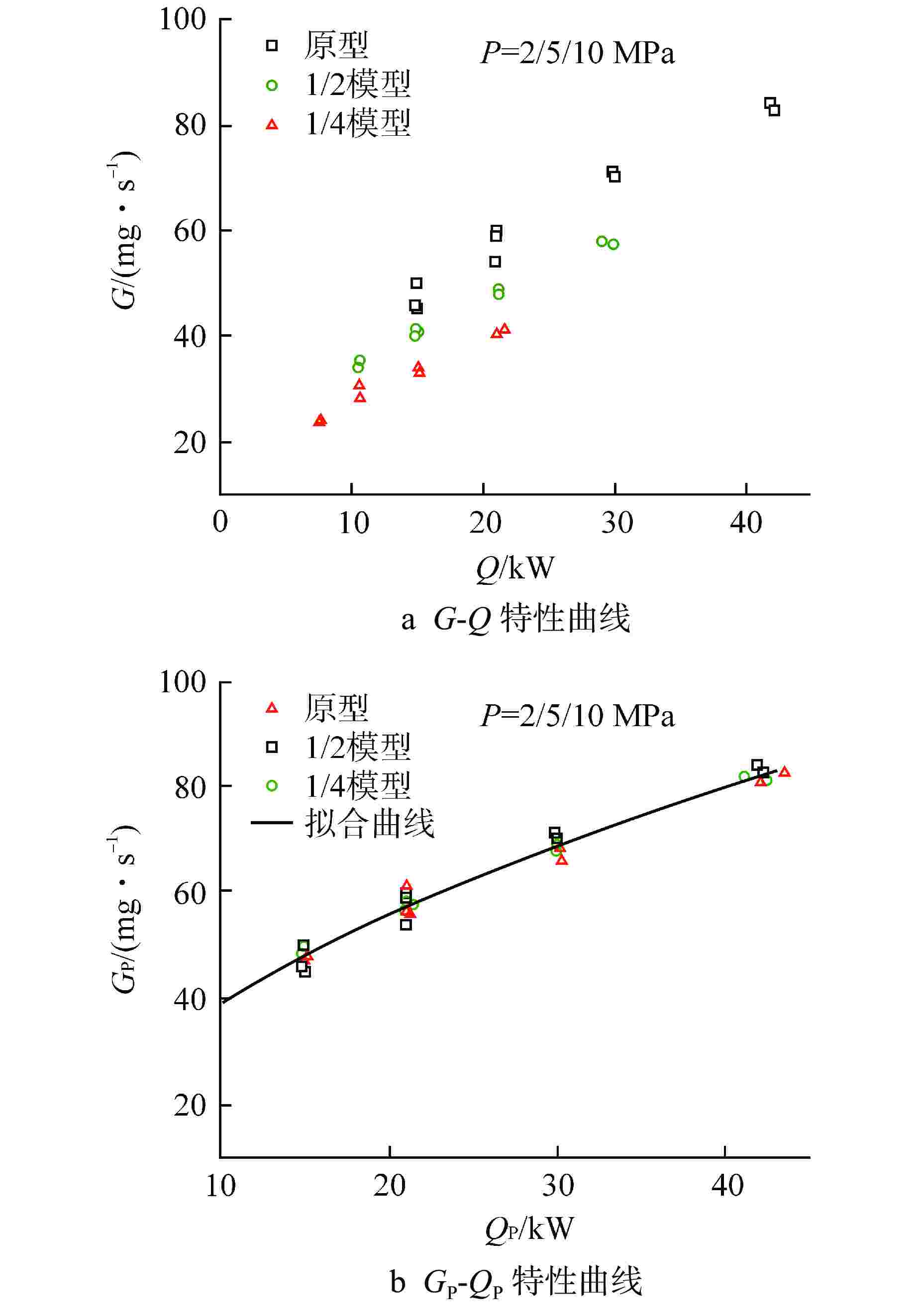
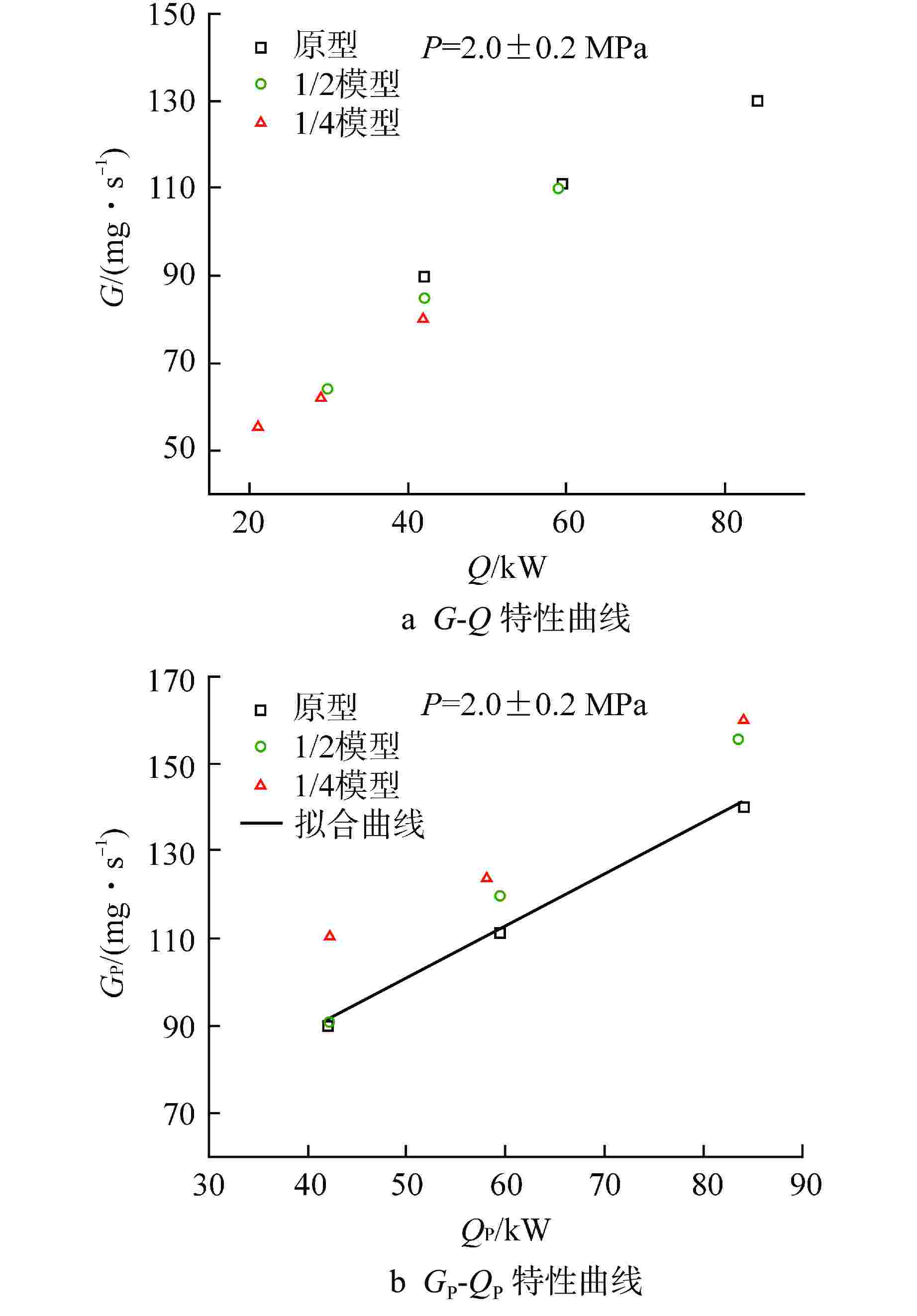
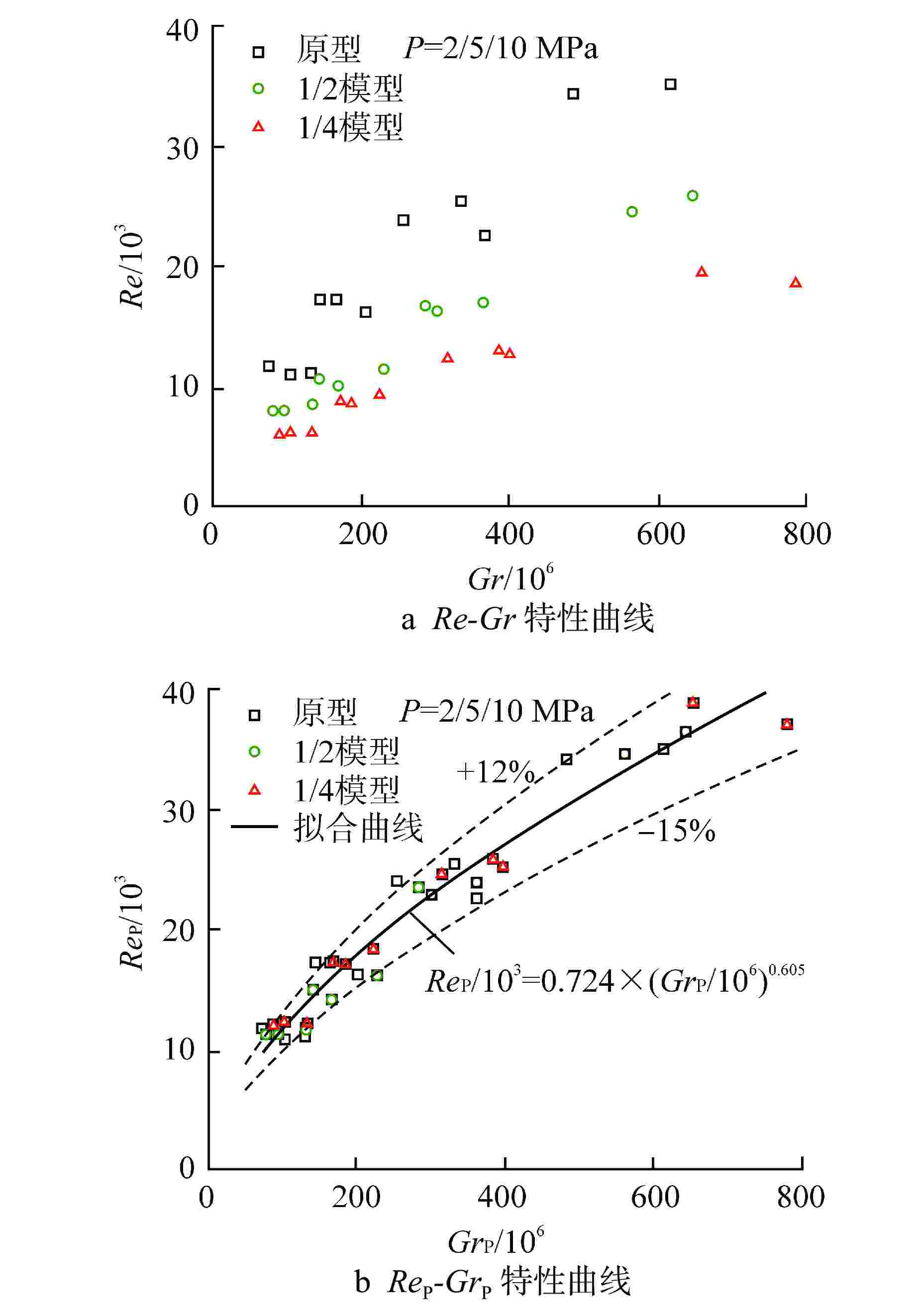
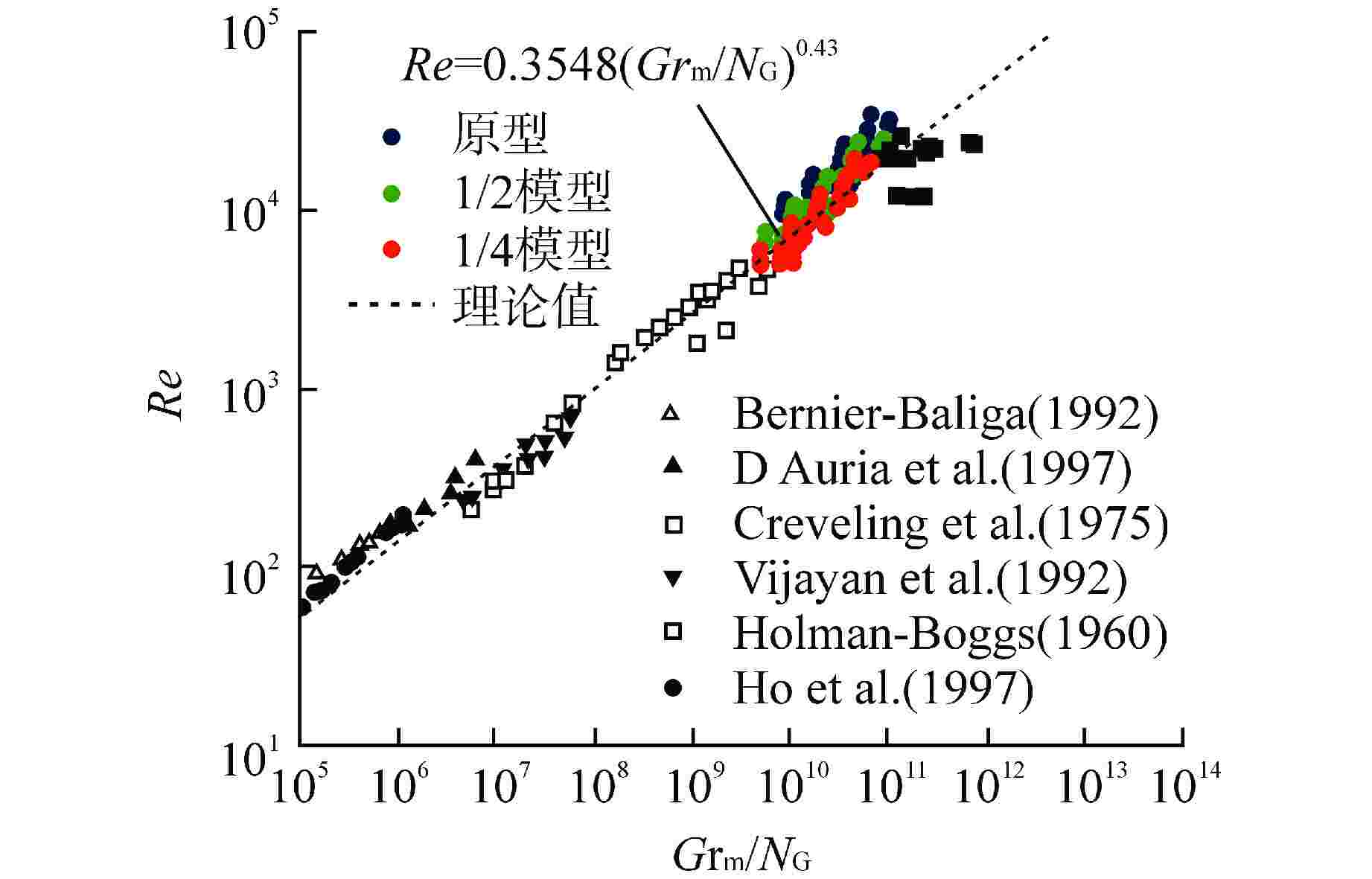
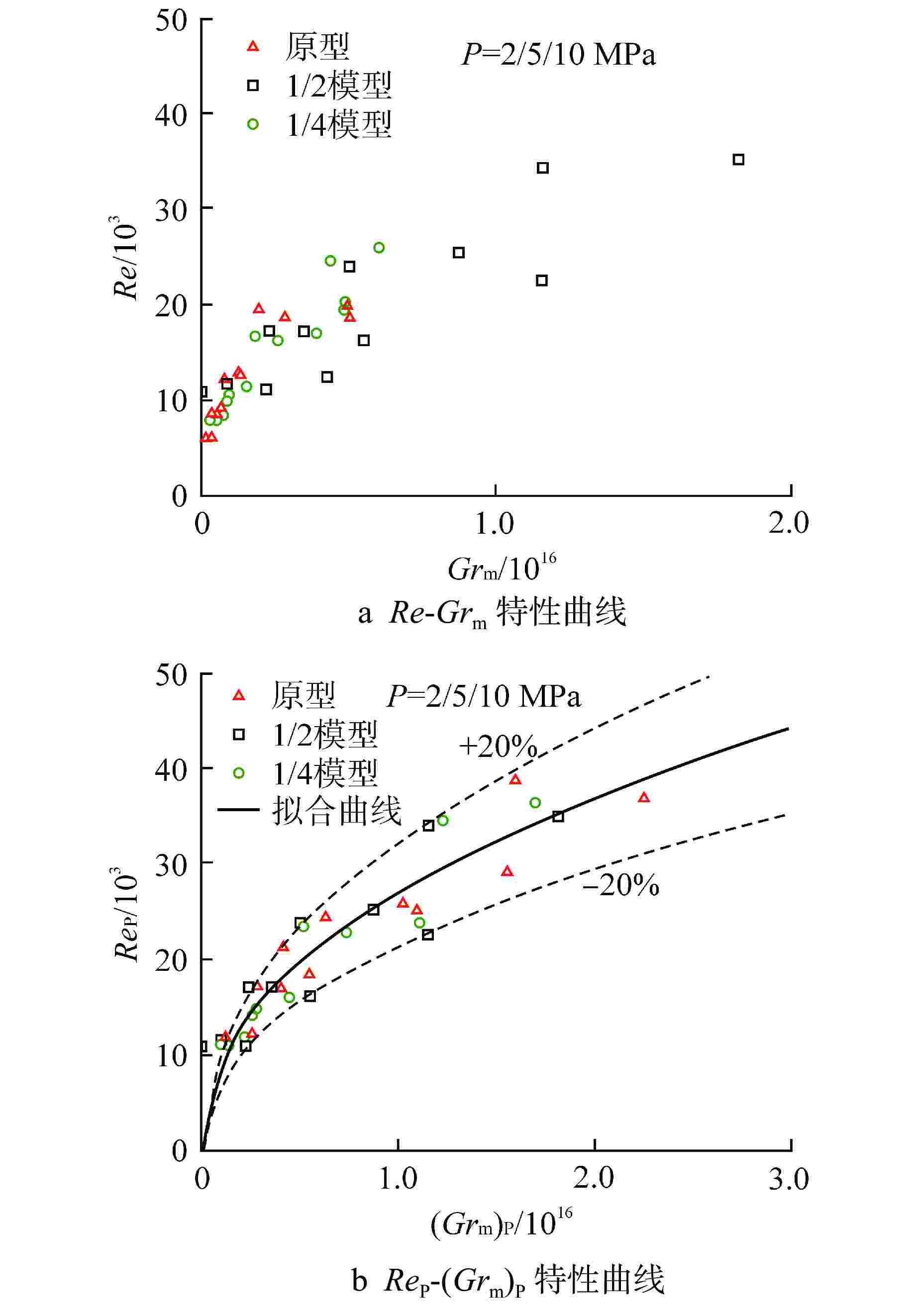
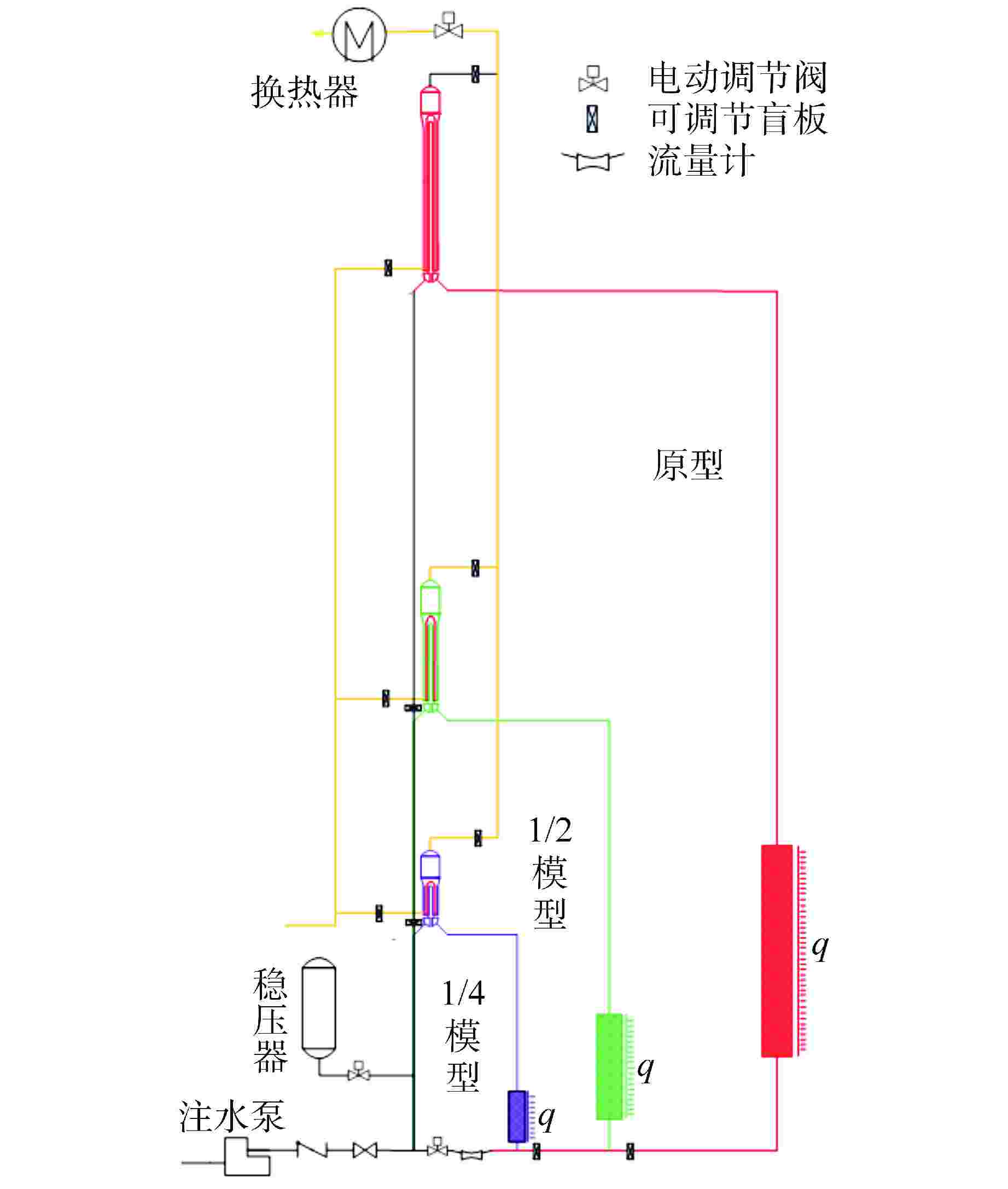
摘要: 基于多级双向比例分析(H2TS)比例模化方法,设计建造了原型、1/2高度比与1/4高度比的模型实验装置,开展了不同加热功率下的自然循环实验,对自然循环特性曲线在降高度比例模化中的失真进行了分析,实验结果表明:自然循环流量、雷诺数、格拉晓夫数、修正格拉晓夫数等表征自然循环主要特征的参数在降高度比例模化后,能够保持自然循环流量-加热功率、雷诺数-格拉晓夫数及雷诺数-修正格拉晓夫数等特征关系曲线不严重失真,原型与模型间的拟合曲线能够形成一对一映射。自然循环特性曲线在降高度比例模化过程中能够较准确复现原型规律,自然循环降高度比例模化实验数据在模拟原型中自然循环特性曲线时的可靠性得到有效验证。Abstract: Based on H2TS (Hierarchical, Two-Tiered Scaling) scaled-down method, three groups of experimental facilities, namely prototype, 1/2 model and 1/4 model, were designed and built. Natural circulation experiments on different heating power were carried out. The distortion of natural circulation characteristic curves in the drop-height scaled-down method was analyzed. The experimental results show that the parameters representing the main characteristics of natural circulation, such as natural circulation flow rate, Reynolds number, Grazoff number and modified Grazoff number, can keep the characteristic curves of natural circulation flow rate-heating power, Reynolds number-Grazoff number and Reynolds number-modified Grazoff number from being seriously distorted after being scaled down, and the fitting curves between the prototype and the model can form a one-to-one mapping. The characteristic curves of natural circulation can accurately reproduce the prototype law in the process of drop-height scaling. The reliability of drop-height scaling experiment simulating the natural circulation characteristic curves in the prototype is effectively verified.
-
Key words:
- Natural circulation /
- Characteristic curves /
- Scaling simulation /
- H2TS /
- Distortion evaluation
-
表 1 等压、同物性模拟准则
Table 1. Scaling Criteria for Isobaric and Homogeneity Simulation
序号 准则 注释 1 $ {\displaystyle \Pi _{\text{R}}} = \dfrac{{g\Delta {\rho _0}L_{{\text{hc}}}^0}}{{{\rho _0}{u_0}^2}} $ Richardson数,表征浮升力与惯性力间关系 2 ${\displaystyle \Pi _{ {\text{F} },i} } = \displaystyle \sum\limits_i {\left( {\dfrac{ {\rho _r^ + } }{ {\rho _i^ + } } } \right)} {\left( {\dfrac{ { {A_r} } }{ { {A_i} } } } \right)^2}{\left( {\dfrac{ {fl} }{ {D_{\rm{e}}} } + k} \right)_i}$ 阻力系数,表征回路的摩擦和形阻 3 $ {\displaystyle \Pi _{{\text{H}},i}} = \dfrac{{{\xi _i}{q_{\text{s}}}l_{{\text{hc}}}^0}}{{{\rho _0}{h_0}{u_0}{a_i}}} $ 热源数,表征热源加热功率对回路焓升的影响 4 $ {\displaystyle \Pi _{{\text{C}},i}} = \dfrac{{{\rho _{\text{s}}}C{v_{\text{s}}}\Delta {T_0}{a_{{\text{s}},i}}}}{{{\rho _0}\Delta {h_{{\text{fg}}}}{a_i}}} $ 热容数,表征结构的热容量对回路焓升的影响 表 2 实验装置主要结构参数
Table 2. Main Structural Parameters of Experimental Facilities
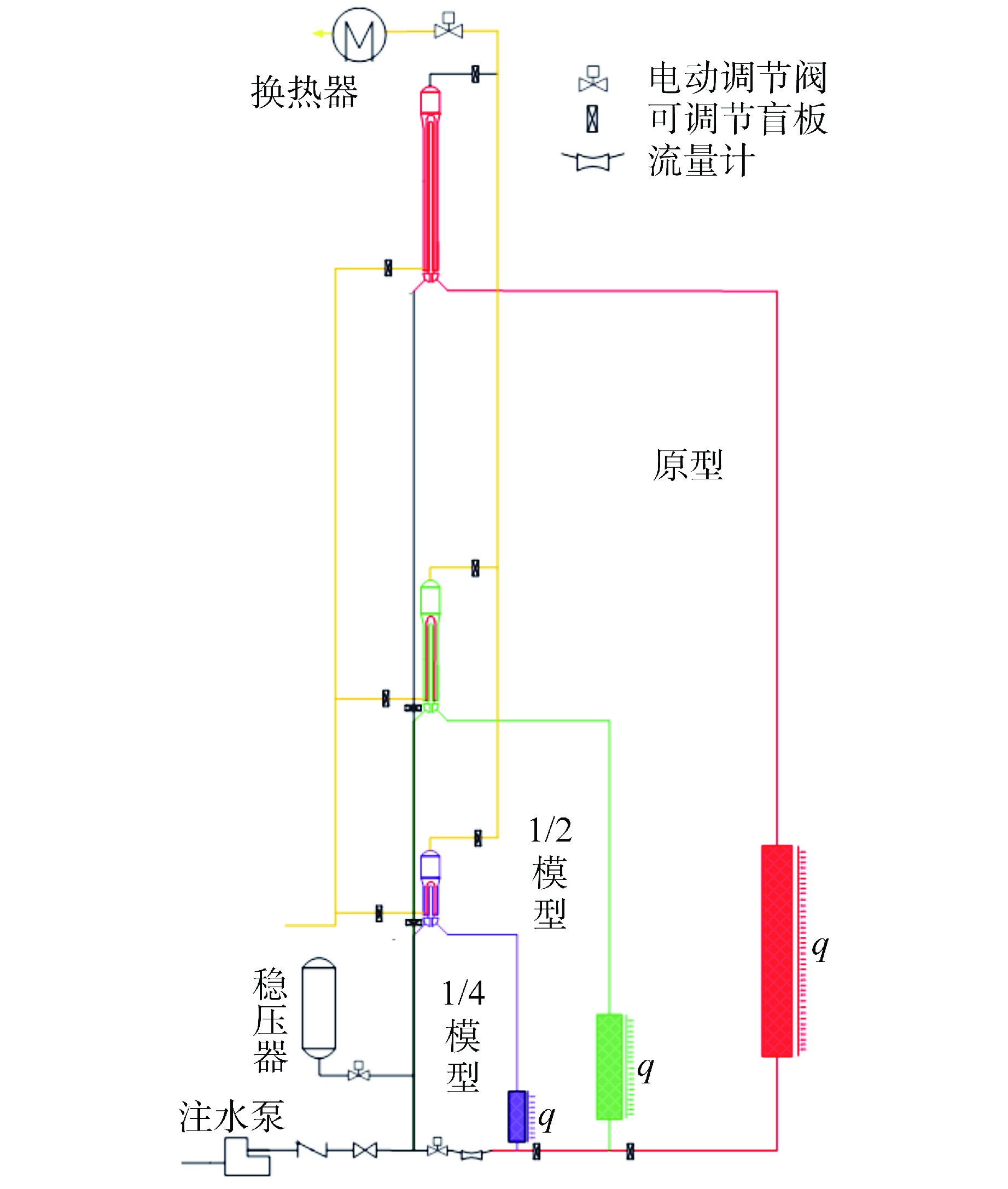
装置 参数 原型 1/2模型 1/4模型 热源 有效加热段高度/mm 3660 1830 915 热源总高度/mm 4634 2804 1889 子通道当量直径/mm 11.8 电加热管规格/mm Φ21×2 承压壳规格/mm Φ255×32.5 热阱 筒体高度/mm 12815 6411 3208 筒体规格/mm Φ114.3×8 传热管高度/m 10605 5282 2611 传热管规格/mm Φ19×2 传热管数目/根 2 一回路管道 冷热芯形位差/m 16 8 4 冷管直径/mm 17.0 冷管长度/m 6 3 1.5 热管直径/mm 18.0 热管长度/m 4.9 2.45 1.225 -
[1] 程诚,文青龙,卢冬华,等. 非能动安全壳冷却系统瞬态特性试验研究[J]. 核动力工程,2017, 38(1): 6-9. doi: 10.13832/j.jnpe.2017.01.0006 [2] 陶俊,程诚,谢小飞,等. 采用分离式热管的非能动安全壳冷却系统研究[J]. 原子能科学技术,2018, 52(8): 1423-1430. [3] WANG S P, LIN Y C, WANG G Y, et al. Experimental investigation on flashing-induced flow instability in a natural circulation scaled-down test facility[J]. Annals of Nuclear Energy, 2022, 171: 109023. doi: 10.1016/j.anucene.2022.109023 [4] LIU L M, PETERSON P, ZHANG D L, et al. Scaling and distortion analysis using a simple natural circulation loop for FHR development[J]. Applied Thermal Engineering, 2020, 168: 114849. doi: 10.1016/j.applthermaleng.2019.114849 [5] ZUBER N, ROHATGI U S, WULFF W, et al. Application of fractional scaling analysis (FSA) to loss of coolant accidents (LOCA): methodology development[J]. Nuclear Engineering and Design, 2007, 237(15-17): 1593-1607. doi: 10.1016/j.nucengdes.2007.01.017 [6] ISHII M, KATAOKA I. Scaling laws for thermal-hydraulic system under single phase and two-phase natural circulation[J]. Nuclear Engineering and Design, 1984, 81(3): 411-425. doi: 10.1016/0029-5493(84)90287-5 [7] KIM H, PARK J P, JANG D, et al. Development of phenomena identification and ranking tables (PIRTs) to implement research reactor-specific capability in SPACE code[J]. Annals of Nuclear Energy, 2020, 138: 107206. doi: 10.1016/j.anucene.2019.107206 [8] LI X B, LI H Y, LIU Y S, et al. Numerical scaling assessment on natural circulation in Core Makeup Tank[J]. Annals of Nuclear Energy, 2020, 140: 107105. doi: 10.1016/j.anucene.2019.107105 [9] 曾未,王杰,黄涛,等. 压水堆大破口失水事故重要现象识别及数值计算不确定性量化分析研究[J]. 核动力工程,2021, 42(1): 198-203. doi: 10.13832/j.jnpe.2021.01.0198 [10] LU D H, XIAO Z J, CHEN B D. A new method to derive one set of scaling criteria for reactor natural circulation at single and two-phase conditions[J]. Nuclear Engineering and Design, 2010, 240(11): 3851-3861. doi: 10.1016/j.nucengdes.2010.08.012 [11] 卢冬华,肖泽军,陈炳德,等. 压水堆自然循环比例模化基本方程及相似准则数的研究[J]. 核动力工程,2009, 30(3): 72-84,94. [12] VIJAYAN P K, MEHTA S K, DATE A W. On the steady-state performance of natural circulation loops[J]. International Journal of Heat and Mass Transfer, 1991, 34(9): 2219-2230. doi: 10.1016/0017-9310(91)90048-J [13] VIJAYAN P K. Experimental observations on the general trends of the steady state and stability behaviour of single-phase natural circulation loops[J]. Nuclear Engineering and Design, 2002, 215(1-2): 139-152. doi: 10.1016/S0029-5493(02)00047-X -





 下载:
下载: