基于阻力分析的矩形通道内弹状流向搅混流转变特性研究

何清澈; 张卢腾; 潘良明; 许汪涛; 闫美月; 孙皖; 马在勇

doi:10.13832/j.jnpe.2023.06.0127

基于阻力分析的矩形通道内弹状流向搅混流转变特性研究

doi: 10.13832/j.jnpe.2023.06.0127

何清澈^{1, 2,},
张卢腾^{1, 2},
潘良明^{1, 2, ,},
许汪涛^{1, 2},
闫美月^{1, 2},
孙皖^{1, 2},
马在勇^{1, 2}

1.
重庆大学低品位能源利用技术及系统教育部重点实验室，重庆，400044
2.
重庆大学核工程与核技术系，重庆，400044

基金项目: 国家自然科学基金联合基金（U21B2059）

详细信息

作者简介:
何清澈（1990—），男，博士研究生，现从事反应堆热工水力实验与理论研究工作，E-mail: leafwilson@foxmail.com

通讯作者:
潘良明，E-mail: cneng@cqu.edu.cn

中图分类号: TL334
计量
- 文章访问数: 74
- HTML全文浏览量: 27
- PDF下载量: 16
- 被引次数: 0
出版历程
- 收稿日期: 2023-02-06
- 修回日期: 2023-03-20
- 网络出版日期: 2023-12-11
- 刊出日期: 2023-12-15

Study on Transition Criteria of Slug Flow to Churn Flow in Vertical Rectangular Channel Based on Characteristics of Interfacial Force

He Qingche^{1, 2
,},
Zhang Luteng^{1, 2},
Pan Liangming^{1, 2
, ,},
Xu Wangtao^{1, 2},
Yan Meiyue^{1, 2},
Sun Wan^{1, 2},
Ma Zaiyong^{1, 2}

1.
Key Laboratory of Low-grade Energy Utilization Technologies and Systems, Ministry of Education, Chongqing University, Chongqing, 400044, China
2.
Department of Nuclear Engineering and Technology, Chongqing University, Chongqing, 400044, China

摘要

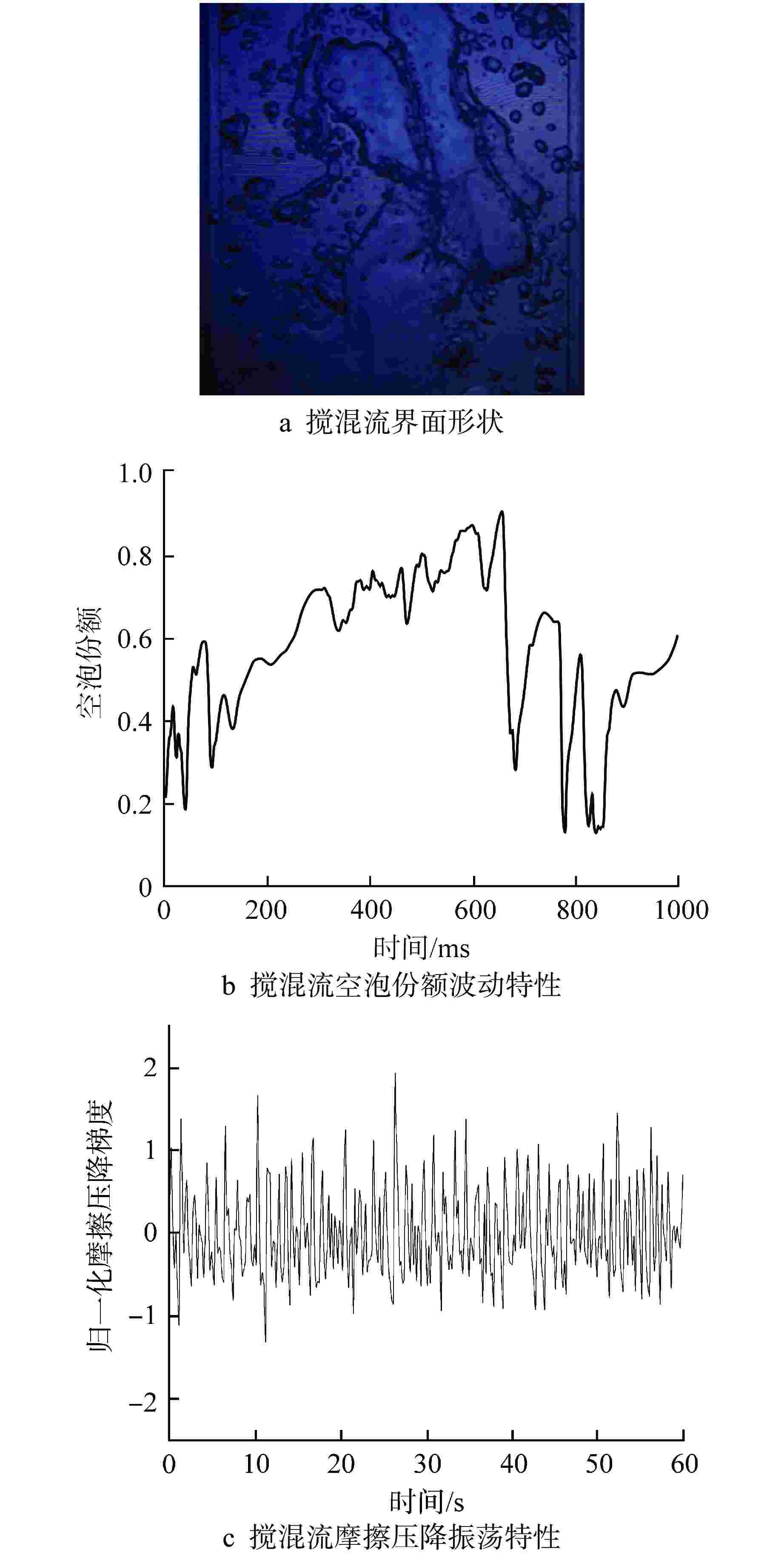
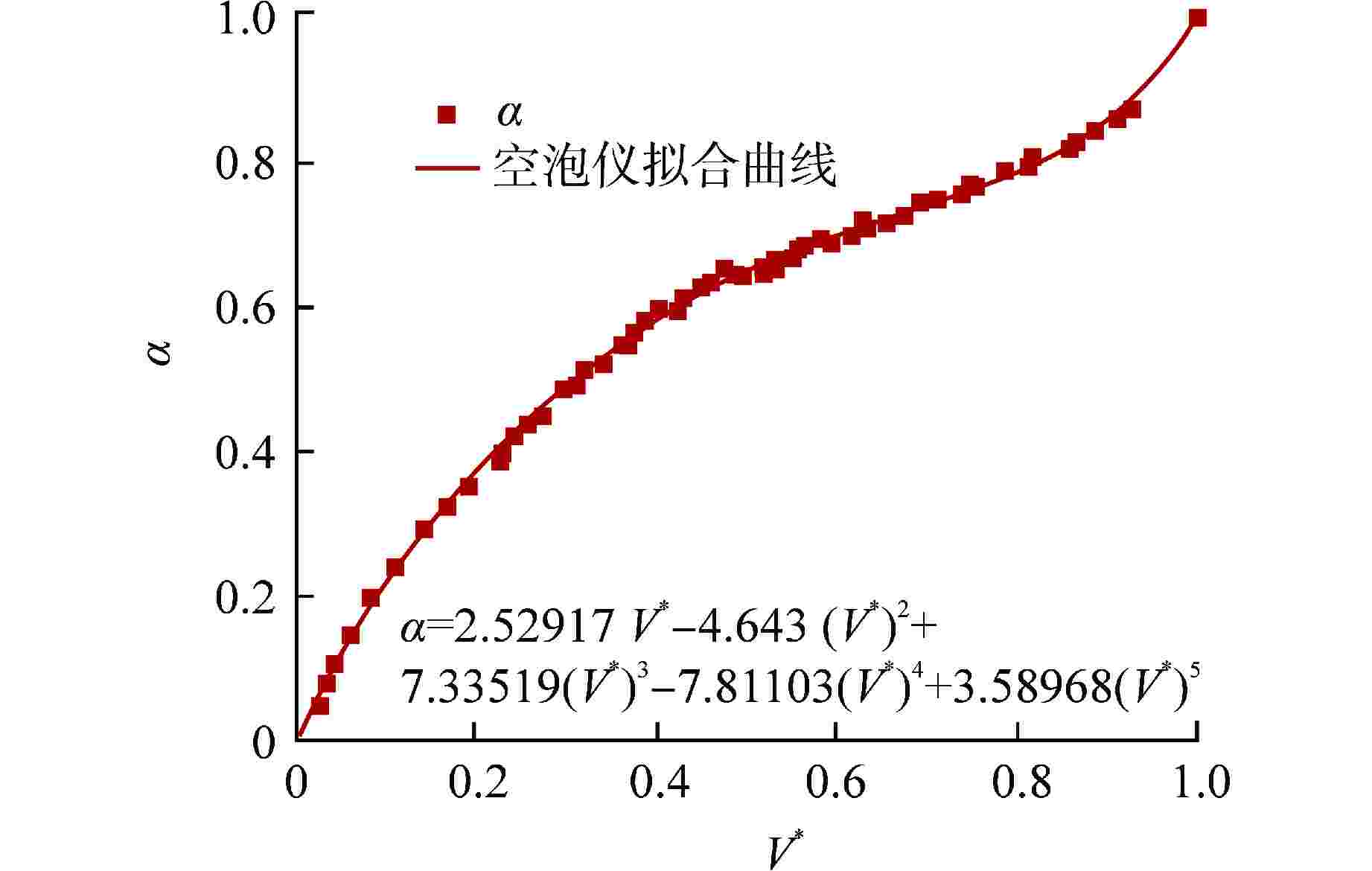
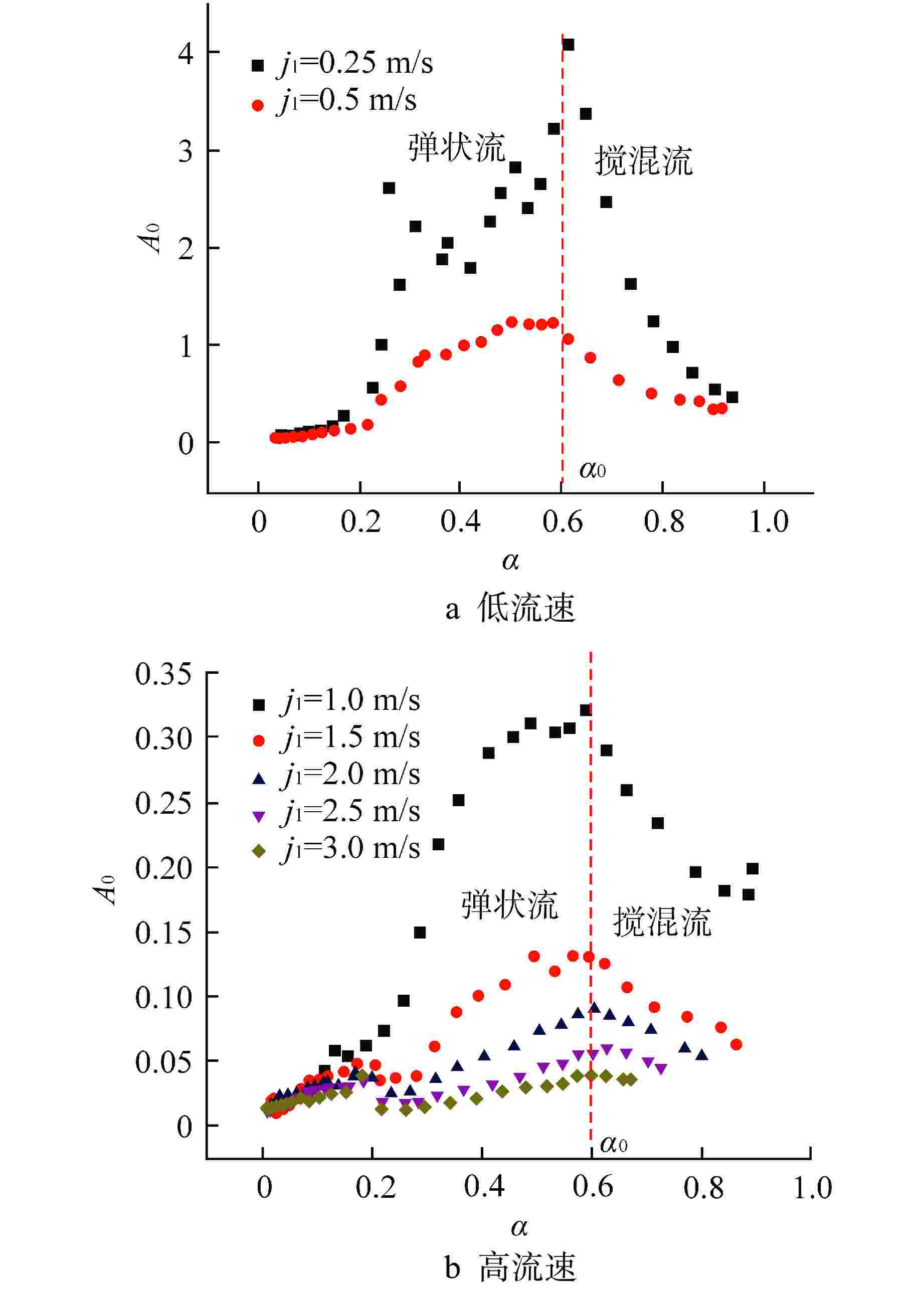
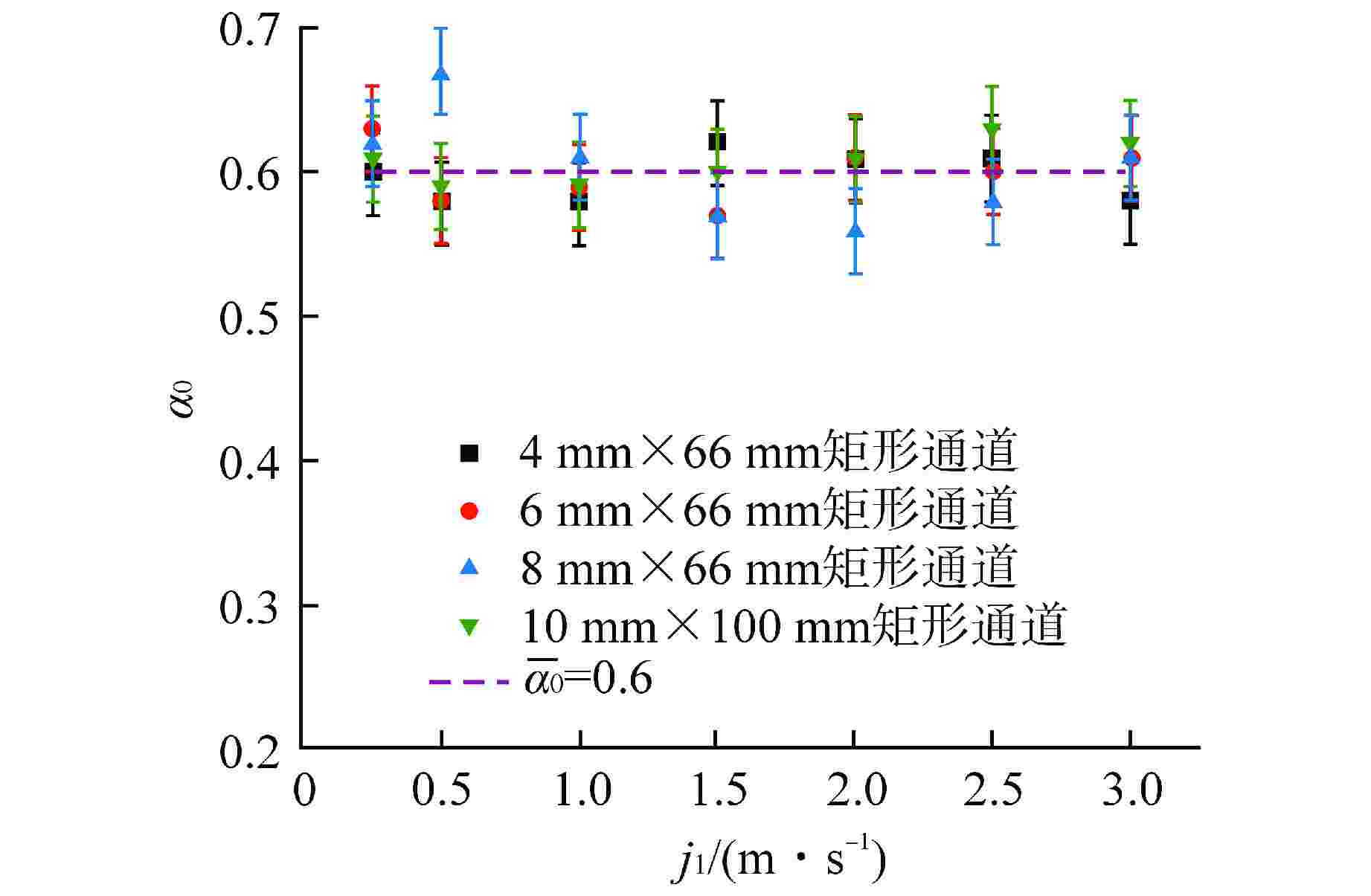
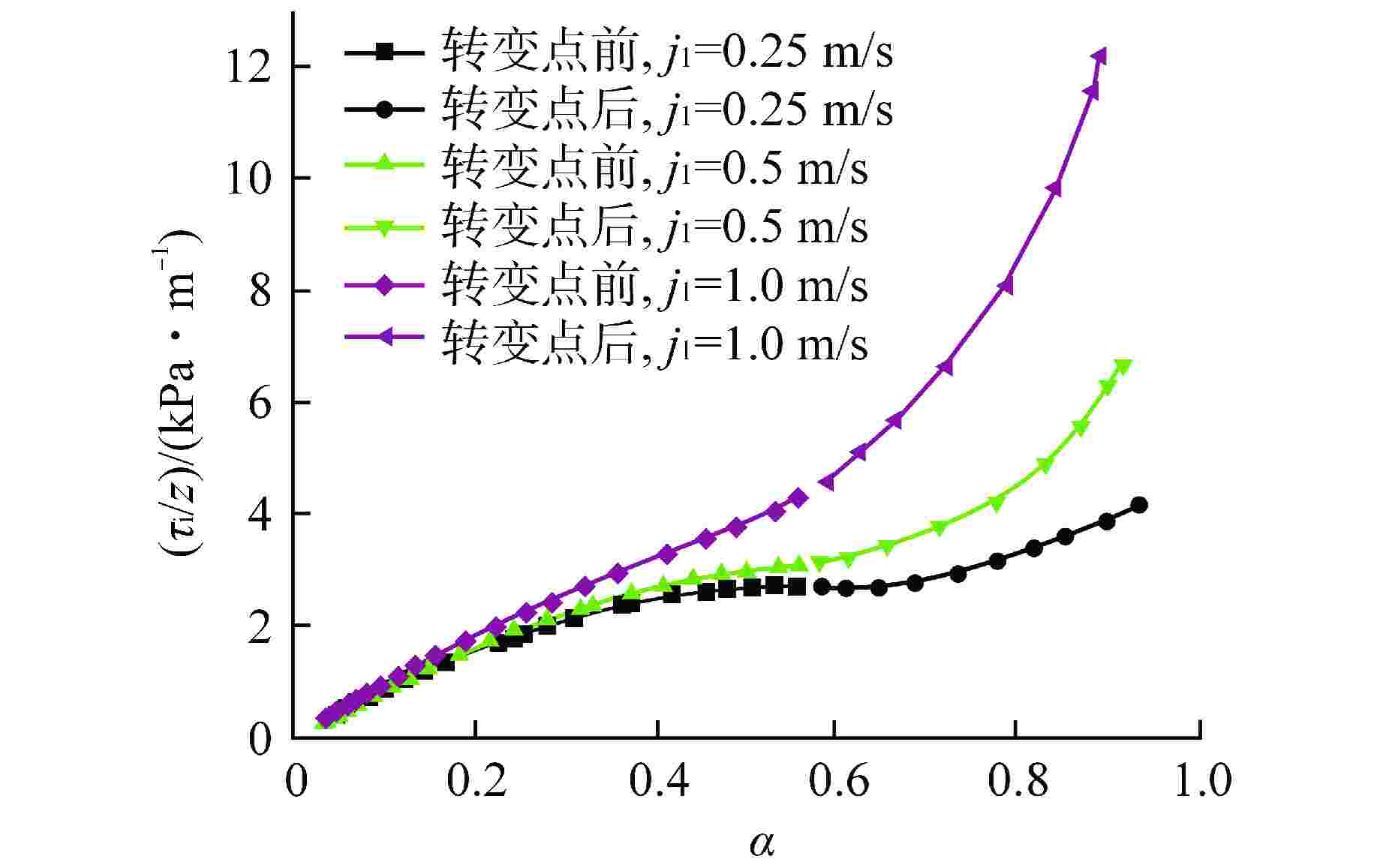
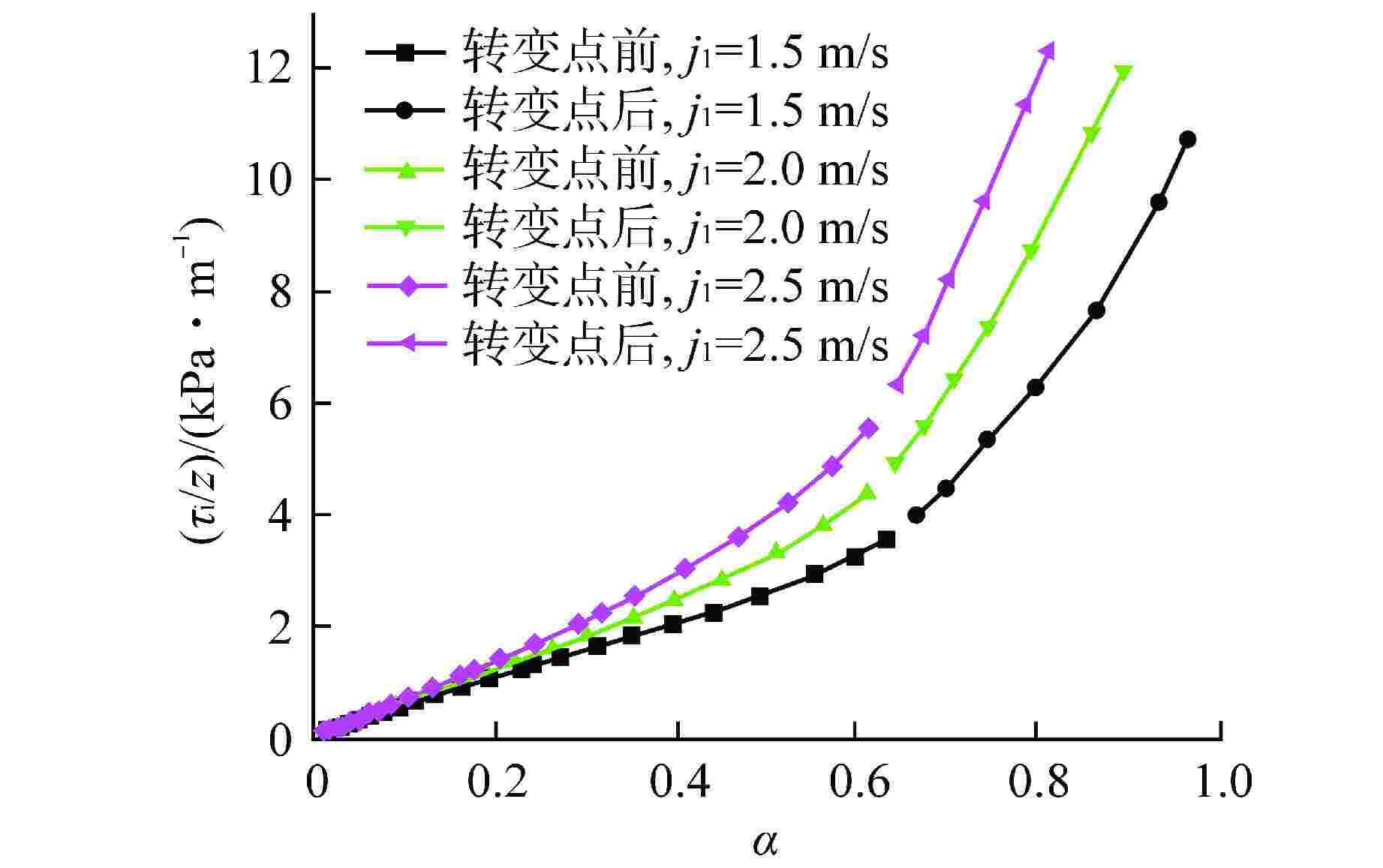
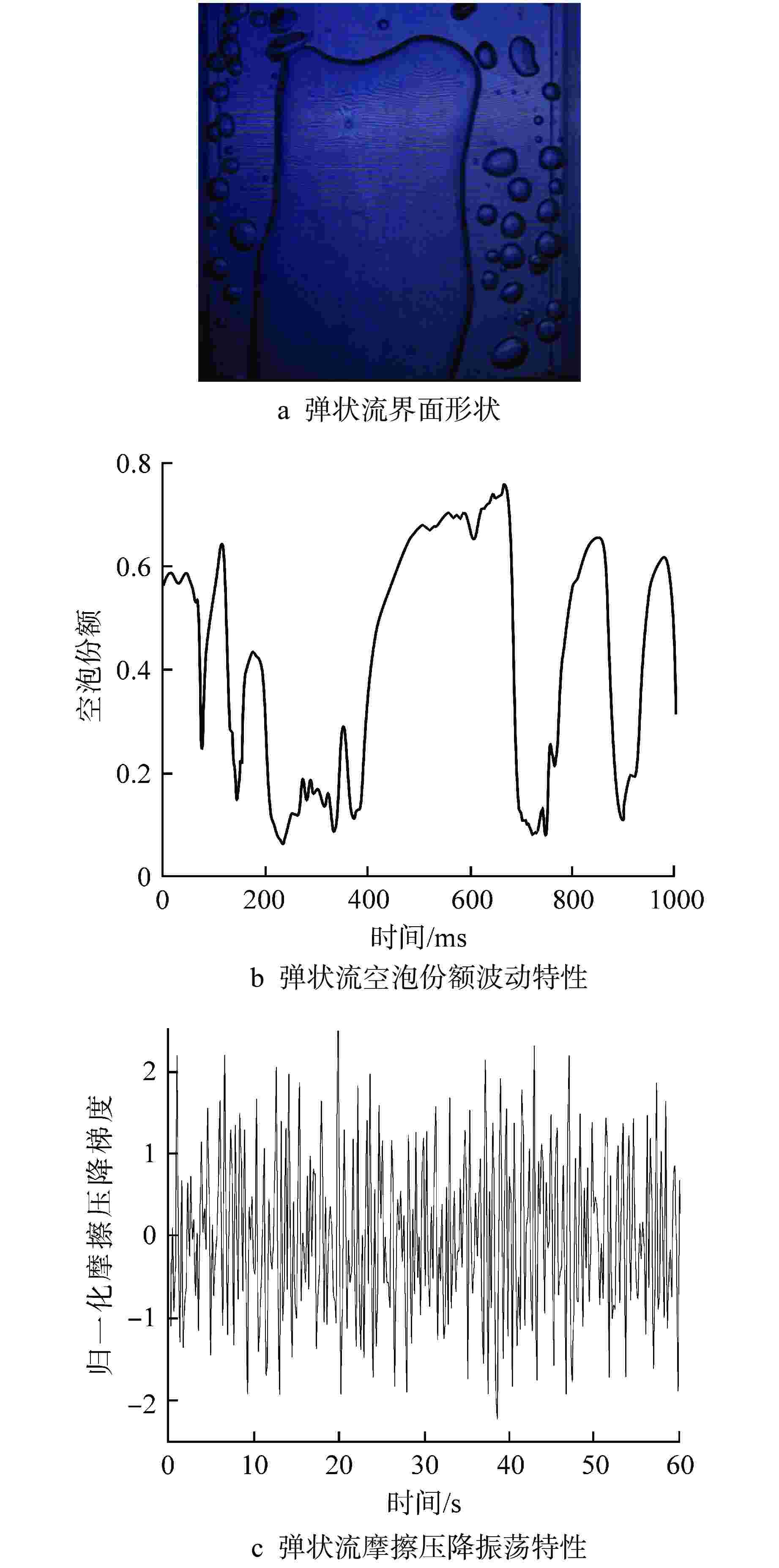
摘要: 确定两相流流型是两相流动相间阻力和传热计算的先决条件，因此不同流型间的过渡准则对反应堆安全分析有十分重要的意义。本文选取了截面尺寸为4 mm×66 mm、6 mm×66 mm、8 mm×66 mm、10 mm×100 mm共4种规格矩形通道开展了空气-水垂直向上两相流动流型实验研究，采用阻抗式空泡仪和差压变送器对截面空泡份额和轴向压降进行测量。研究结果表明，在相同液相表观流速下，随空泡份额的增加，两相摩擦压降波动的振幅在弹状流向搅混流转变时达到最大；在空泡份额为0.6时，开始发生弹状流向搅混流转变。实验结果还显示，在流型转变点后相间阻力梯度随空泡份额而快速增加，在力学特性层面，可以采用相间阻力梯度作为弹状流向搅混流转变的判据。
- 两相流 /
- 矩形通道 /
- 流型 /
- 空泡份额 /
- 相间阻力
Abstract: The transitional criteria between different flow regimes is of great significance to the safety analysis of nuclear reactor operation, because determining the flow regime of two-phase flow is a prerequisite for calculating the interfacial force and heat transfer of two-phase flow. In this study, four types of rectangular channels with cross-sectional size of 4×66 mm, 6×66 mm, 8×66 mm and 10×100 mm were employed to conduct the research on air-water upward flow in rectangular channels, and the void fraction and pressure drop were measured by impedance void meter and differential pressure gauge respectively. The results show that, at the constant superficial liquid velocity, the amplitude of frictional pressure drop reaches the maximum value at the transitional point of slug flow to churn flow, and when the void fraction is 0.6, the transition from slug flow to churn flow begins. The experimental results also show that the interfacial force gradient increases rapidly with the void fraction after the flow regime transition point, and the interfacial force gradient can be used as the criterion for the transition from slug flow to churn flow in terms of mechanical characteristics..
- Two-phase flow /
- Rectangular channel /
- Flow regime /
- Void fraction /
- Interfacial force

HTML全文

图 1 弹状流特性示意图

Figure 1. Schematic Diagram of Slug Flow Characteristics

下载: 全尺寸图片幻灯片

图 2 搅混流特性示意图

Figure 2. Schematic Diagram of Churn Flow Characteristics

下载: 全尺寸图片幻灯片

图 3 气-水两相流实验回路

Figure 3. Experimental Loop of Air-water Two-phase Flow

下载: 全尺寸图片幻灯片

图 4 空泡仪标定曲线图

Figure 4. Calibration Curve of Impedance Void Meter

下载: 全尺寸图片幻灯片

图 5 最大摩擦压降振荡幅值随空泡份额变化

Figure 5. Variation of Maximum Amplitude of Frictional Pressure Drop with Void Fraction

下载: 全尺寸图片幻灯片

图 6 不同条件下压力振荡极值点对应的空泡份额

Figure 6. Void Fraction Corresponding to the Peak of Maximum Amplitude under Different Conditions

下载: 全尺寸图片幻灯片

图 7 低液相表观流速条件下，相间阻力随空泡份额变化图

Figure 7. Relationship between Interfacial Force and Void Fraction at Low Liquid Flow Rate

下载: 全尺寸图片幻灯片

图 8 高液相表观流速条件下，相间阻力随空泡份额变化图

Figure 8. Relationship between Interfacial Force and Void Fraction at High Liquid Flow Rate

下载: 全尺寸图片幻灯片

表 1 四种矩形通道特征尺寸表

Table 1. Dimension of Four Types of Rectangular Channels

窄缝 G/mm	宽度 W/mm	水力直径 D_h/mm	长宽比 (W/G)	测量长度 ∆z/mm
4	66	7.54	16.5	556
6	66	11.00	11	556
8	66	14.27	8.25	607
10	100	18.20	10	895

下载: 导出CSV

参考文献(12)

[1]	任全耀. 棒束通道内相态分布特性及影响机制研究[D]. 重庆: 重庆大学, 2018.
[2]	PAN L M, ZHANG M H, JU P, et al. Vertical co-current two-phase flow regime identification using fuzzy C-means clustering algorithm and Relief attribute weighting technique[J]. International Journal of Heat and Mass Transfer, 2016, 95: 393-404. doi: 10.1016/j.ijheatmasstransfer.2015.11.081
[3]	MI Y, ISHII M, TSOUKALAS L H. Vertical two-phase flow identification using advanced instrumentation and neural networks[J]. Nuclear Engineering and Design, 1998, 184(2-3): 409-420. doi: 10.1016/S0029-5493(98)00212-X
[4]	CHALGERI V S, JEONG J H. Flow regime identification and classification based on void fraction and differential pressure of vertical two-phase flow in rectangular channel[J]. International Journal of Heat and Mass Transfer, 2019, 132: 802-816. doi: 10.1016/j.ijheatmasstransfer.2018.12.015
[5]	MISHIMA K, HIBIKI T. Some characteristics of air-water two-phase flow in small diameter vertical tubes[J]. International Journal of Multiphase Flow, 1996, 22(4): 703-712. doi: 10.1016/0301-9322(96)00010-9
[6]	XING D C, YAN C Q, MA X G, et al. Effects of void fraction correlations on pressure gradient separation of air–water two-phase flow in vertical mini rectangular ducts[J]. Progress in Nuclear Energy, 2014, 70: 84-90. doi: 10.1016/j.pnucene.2013.08.003
[7]	MISHIMA K, HIBIKI T, NISHIHARA H. Some characteristics of gas-liquid flow in narrow rectangular ducts[J]. International Journal of Multiphase Flow, 1993, 19(1): 115-124. doi: 10.1016/0301-9322(93)90027-R
[8]	XU J L, CHENG P, ZHAO T S. Gas–liquid two-phase flow regimes in rectangular channels with mini/micro gaps[J]. International Journal of Multiphase Flow, 1999, 25(3): 411-432. doi: 10.1016/S0301-9322(98)00057-3
[9]	WILMARTH T, ISHII M. Two-phase flow regimes in narrow rectangular vertical and horizontal channels[J]. International Journal of Heat and Mass Transfer, 1994, 37(12): 1749-1758. doi: 10.1016/0017-9310(94)90064-7
[10]	HIBIKI T, MISHIMA K. Flow regime transition criteria for upward two-phase flow in vertical narrow rectangular channels[J]. Nuclear Engineering and Design, 2001, 203(2-3): 117-131. doi: 10.1016/S0029-5493(00)00306-X
[11]	TAITEL Y, BARNEA D, DUKLER A E. Modelling flow pattern transitions for steady upward gas-liquid flow in vertical tubes[J]. AIChE Journal, 1980, 26(3): 345-354. doi: 10.1002/aic.690260304
[12]	MISHIMA K, ISHII M. Flow regime transition criteria for upward two-phase flow in vertical tubes[J]. International Journal of Heat and Mass Transfer, 1984, 27(5): 723-737. doi: 10.1016/0017-9310(84)90142-X