Study on CHF Relational Expression Development Based on High-precision Subchannel Code
-
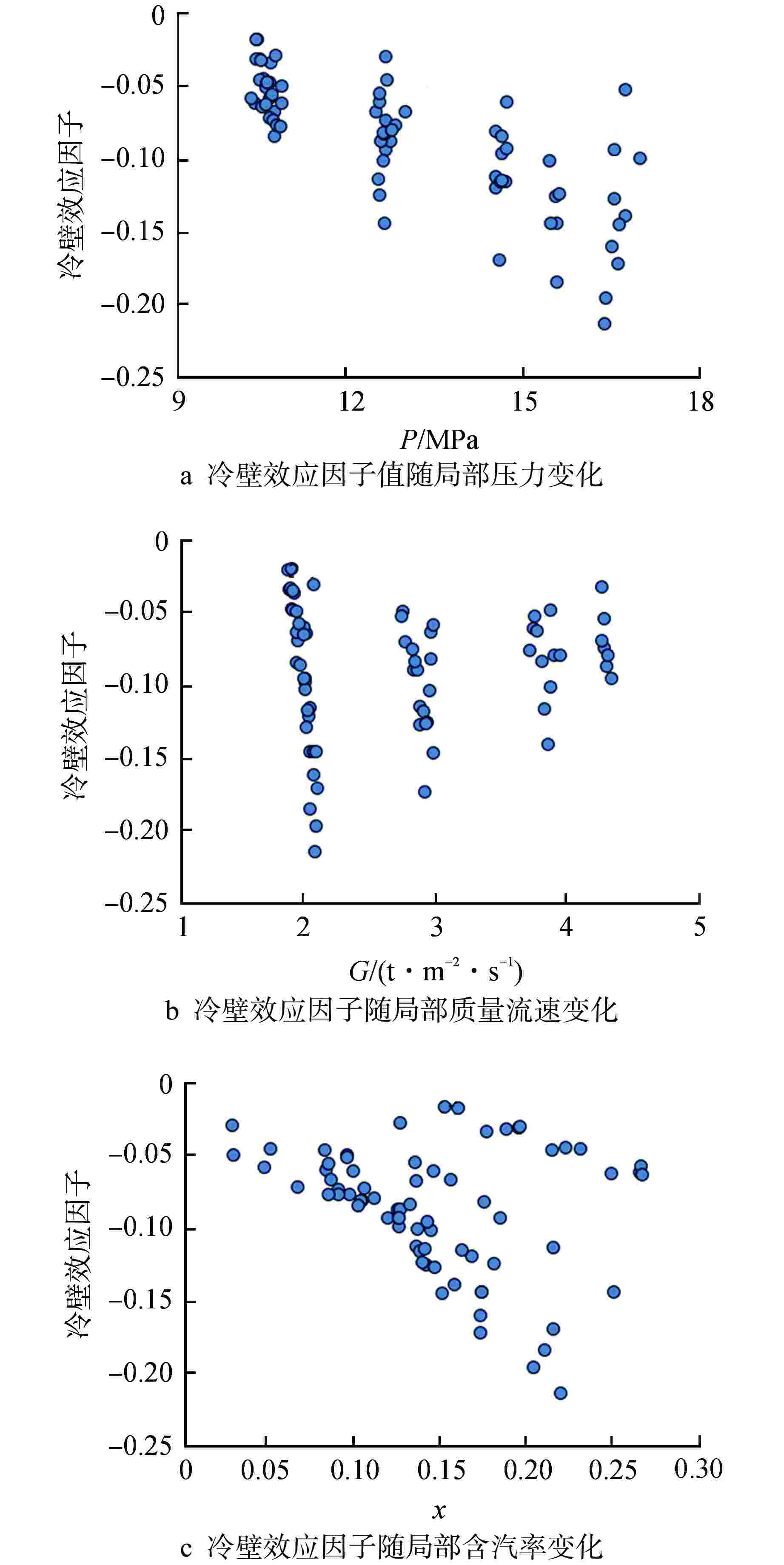
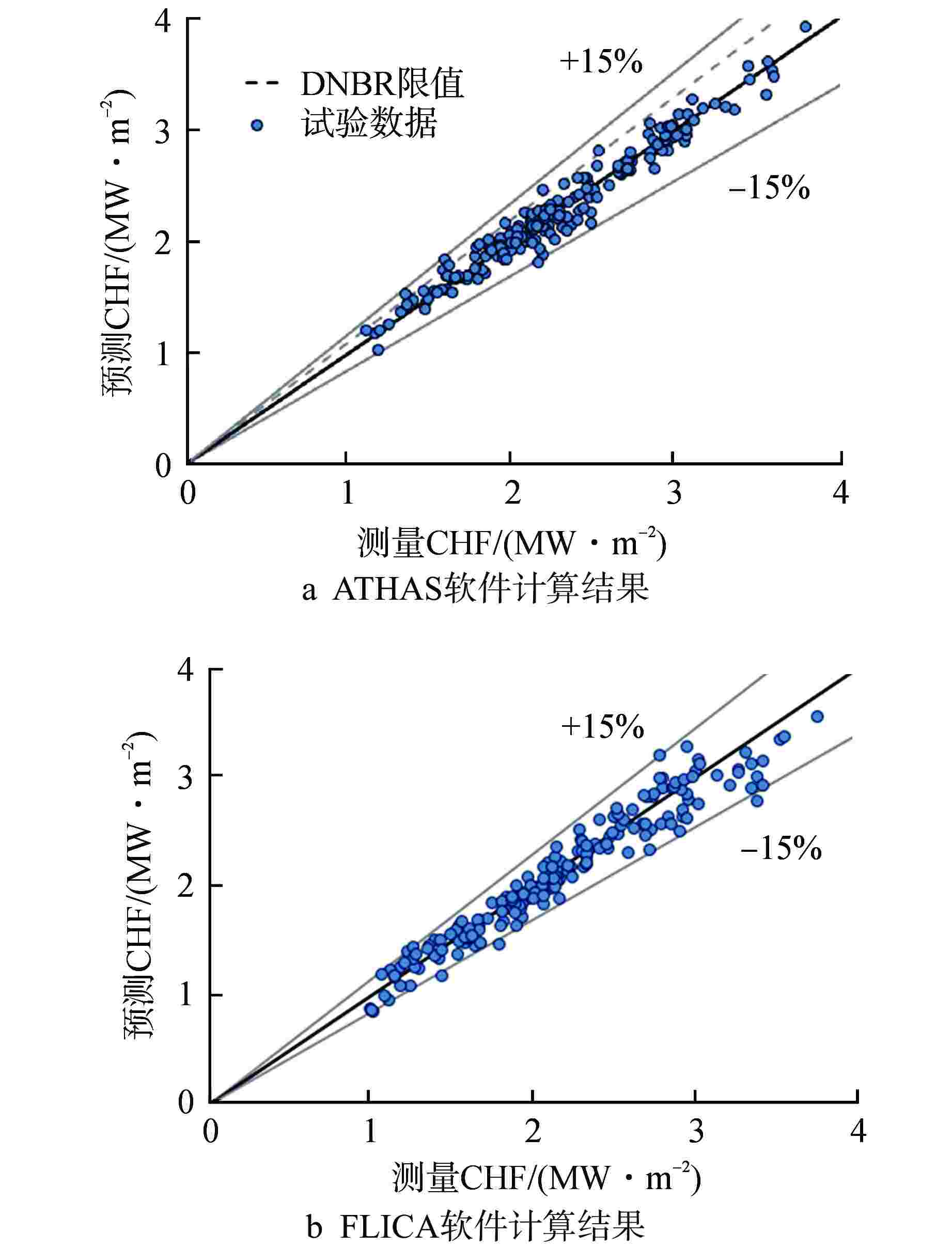
摘要: 采用非均匀加热典型栅元和导向管栅元三组临界热流密度（CHF）试验数据，利用高精度子通道分析软件ATHAS获得局部参数，完成适用于燃料组件偏离泡核沸腾比（DNBR）分析的CHF关系式开发，得到导向管冷壁效应因子和DNBR限值；并与采用FLICA软件开发的关系式结果进行对比，结果表明，ATHAS软件开发的关系式计算得到的DNBR限值更低，且对烧毁（BO）点轴向位置和CHF的预测率更高。
-
关键词:
- ATHAS软件 /
- 临界热流密度(CHF) /
- 偏离泡核沸腾比(DNBR)
Abstract: Three groups of CHF test data of non-uniform heating typical grid and guide tube grid were adopted, and the local parameters were obtained by using high precision subchannel code ATHAS. The development of CHF relation suitable for the analysis of fuel assembly deviation from nucleate boiling ratio (DNBR) was completed, and the cold wall effect factor and DNBR limit of guide tube were obtained. Compared with the results of the correlation developed by FLICA, the DNBR limit calculated by the relationship developed by ATHAS is lower, and the prediction rate of axial position of burn-out (BO) point and CHF is higher. -
图 1 轴向非均匀加热CHF试验件示意图
Figure 1. Schematic Diagram of CHF Test Piece with Axial Non-uniform Heating
图 4 导向管冷壁效应因子随局部参数的变化趋势
Figure 4. Variation Trend of Cold Wall Factor of Guide Tube with Local Parameters
图 5 所有数据M/P值随局部参数的变化趋势
Figure 5. Variation Trend of M/P Ratio of All Test Data with Local Conditions
图 6 所有试验数据P-M分布
Figure 6. Predicted CHF as a Function of Measured CHF of All Test Data
表 1 CHF关系式开发的试验数据
Table 1. Test Data for CHF Correlation Development
试验件编号 栅元类型 数据量 1 典型 58 2 导向管 71 3 典型 59 
下载: 导出CSV
表 2 试验数据工况
Table 2. Test Data Condition
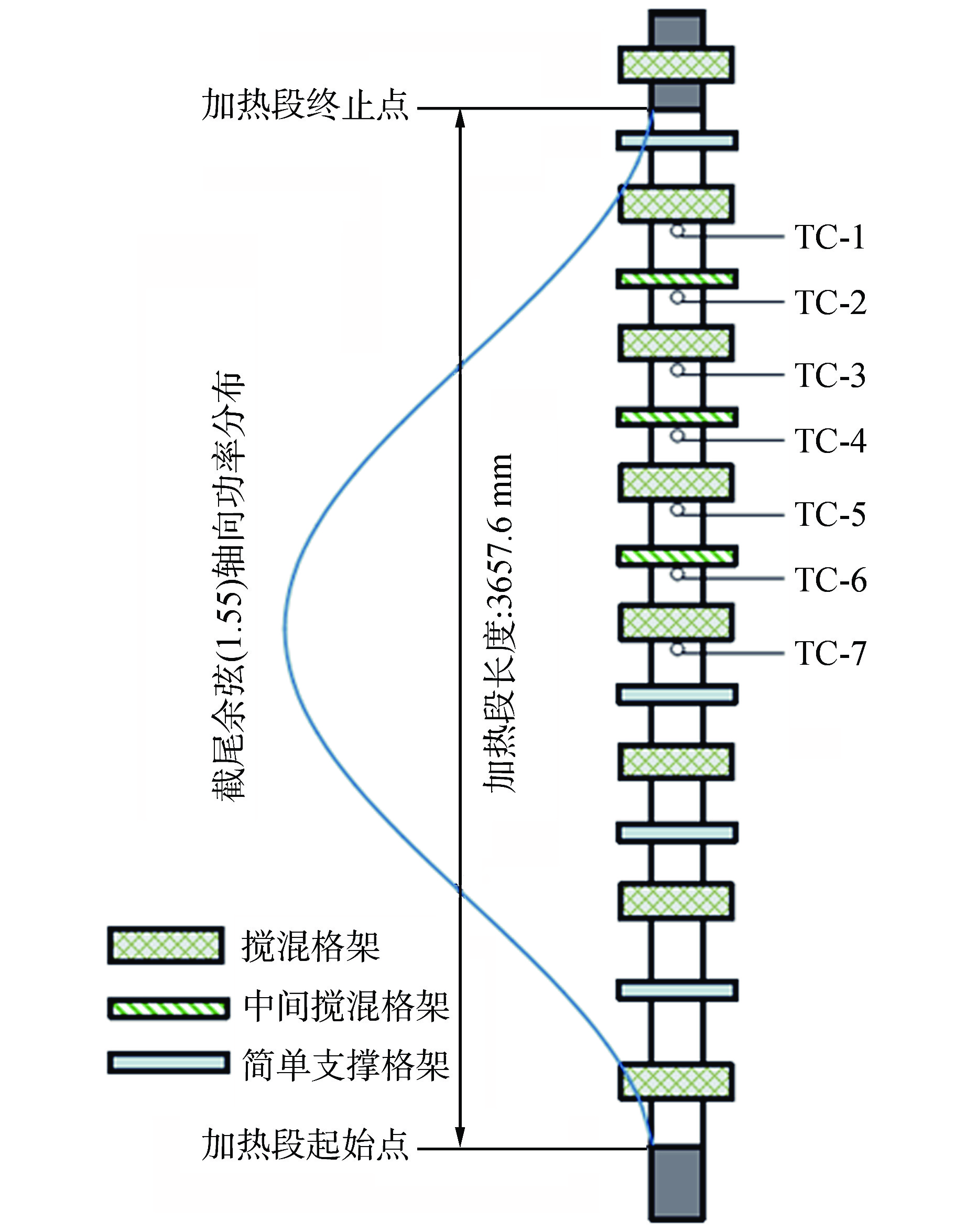
参数名 参数值范围 出口压力/MPa 9.84~16.86 质量流速/(t·m−2·s−1) 1.36~4.57 进口温度/℃ 152.76~301.22 格架跨距/m 0.251~0.522
下载: 导出CSV
表 4 CHF关系式中系数
Table 4. Cofficients in CHF Formula
系数 拟合值 系数 拟合值 a1 23.30 c1 −5.23 a2 −3.11 c2 −7.52 a3 −4.72 c3 3.35 a4 −29.00 d2 −3.09 a5 5.63 d3 0.11 a6 9.71 e1 0.16 a7 −3.79 e2 −4.24 b1 0.44 e3 0.36 b2 5.60 e4 −0.57 b3 17.91 e5 3.99 b4 −1.73
下载: 导出CSV
表 5 M/P数据的统计学计算结果
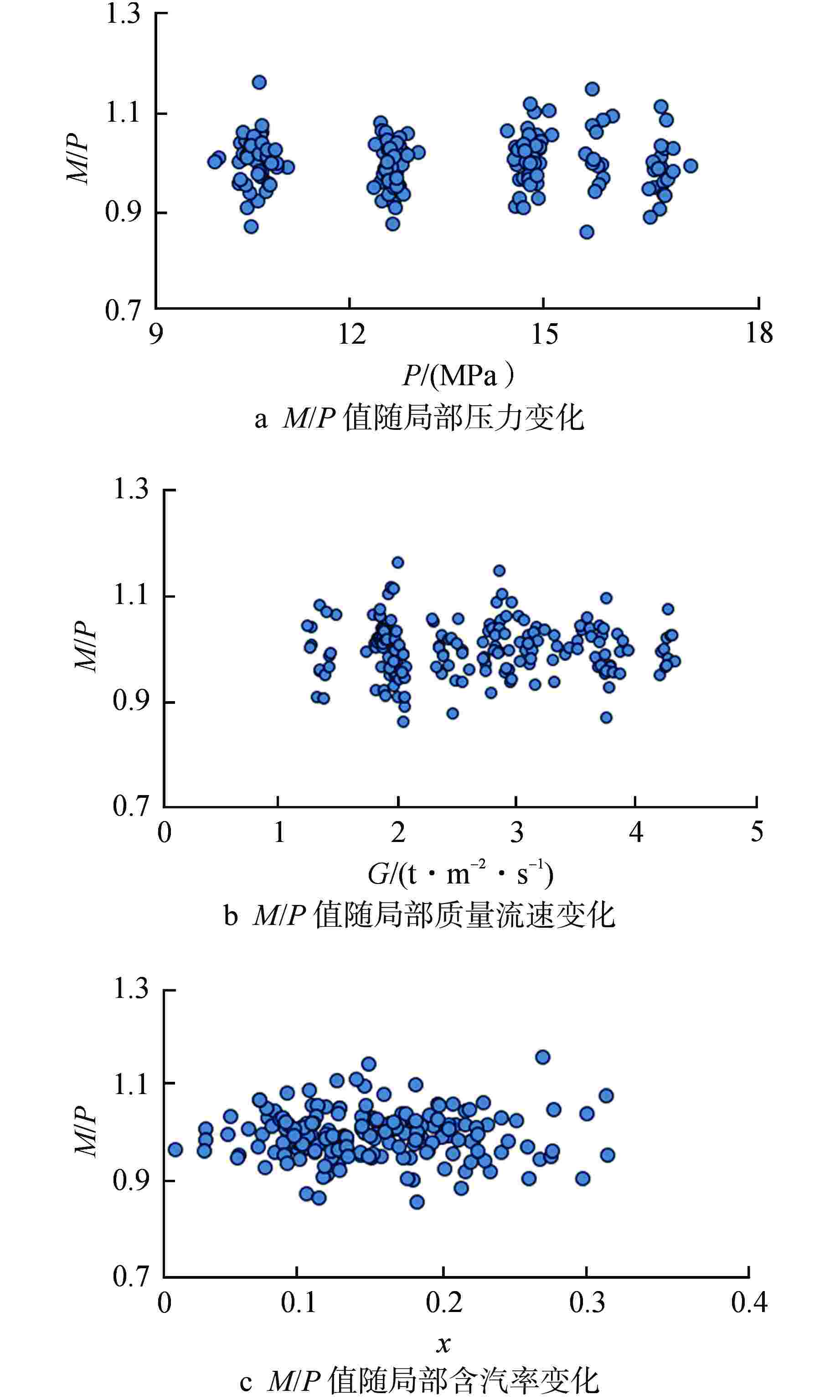
Table 5. Statistic Results of M/P Data
分组 数据点 M/P均值（最小DNBR点） M/P标准差（最小DNBR点） T-1 58 1.0004 0.0485 T-2 71 0.9991 0.0513 T-3 59 1.0005 0.0502 总计 188 1.0000 0.0498
下载: 导出CSV
表 6 试验数据总体检验
Table 6. Overall Test of Experimental Data
分组 正态检验P值 方差检验P值 均值检验P值 T-1 0.7527 0.9071 0.9834 T-2 0.1533 T-3 0.6320
下载: 导出CSV
表 7 采用ATHAS和FLICA软件开发关系式的结果对比
Table 7. Comparison of the Results of Relational Expressions Developed by ATHAS and FLICA
软件名 ATHAS FLICA M/P均值 1.000 1.030 M/P标准偏差 0.0498 0.072 DNBR限值 1.102 1.113 轴向预测率/% 61.7 59.6 轴向位置预测率/% 94.1 83
下载: 导出CSV
-
[1] TONG L S, TANG Y S. Boiling heat transfer and two-phase flow[M]. 2nd ed. Washington: Taylor & Francis, 1997: 366-401. [2] TONG L S. Boiling crisis and critical heat flux: NSA-26-055179[R]. Pittsburgh: Westinghouse Electric Corp., 1972. [3] CHANG S H, BAEK W P. Understanding, predicting, and enhancing critical heat flux[C]//Proceedings of the 10th International Topical Meeting on Nuclear Reactor Thermal Hydraulics, Seoul, 2003. [4] 张玉相,席炎炎,庞铮铮,等. CHF关系式开发与DNBR限值确定方法研究[J]. 核动力工程,2016, 37(5): 130-134. [5] REDDY D G, FIGHETTI C F. Parametric study of CHF data. Volume 2. A generalized subchannel CHF correlation for PWR and BWR fuel assemblies. Final report: EPRI-NP-2609-Vol. 2[R]. New York: Columbia University, 1983. [6] DAVIDSON S L, KRAMER W R. Reference core report VANTAGE 5 fuel assembly: WCAP-10445-NP-A[R]. Pittsburgh: Westing-House Electric Corp. , 1985. [7] 柴国旱,王小海,陈召林,等. 对临界热流密度计算关系式FC-2000的审评[J]. 核动力工程,2003, 12(S2): 84-87,96. [8] 蒋理,单建强. 高精度子通道分析方法应用于PSBT棒束试验研究[C]//中国核学会反应堆热工流体力学分会第一届学术年会暨中核核反应堆热工水力技术重点试验室2021年学术年会, 重庆,2021. [9] NINOKATA H, EFTHIMIADIS A, TODREAS N E. Distributed resistance modeling of wire-wrapped rod bundles[J]. Nuclear Engineering and Design, 1987, 104(1): 93-102. doi: 10.1016/0029-5493(87)90306-2 [10] CARLUCCI L N, HAMMOUDA N, ROWE D S. Two-phase turbulent mixing and buoyancy drift in rod bundles[J]. Nuclear Engineering and Design, 2004, 227(1): 65-84. doi: 10.1016/j.nucengdes.2003.08.003 [11] DITTUS F W, BOELTER L M K. Heat transfer in automobile radiators of the tubular type[M]. Berkley: University of California, 1930: 443-461. [12] YANG B W, ANGLART H, HAN B, et al. Progress in rod bundle CHF in the past 40 years[J]. Nuclear Engineering and Design, 2021, 376: 111076. doi: 10.1016/j.nucengdes.2021.111076 [13] 李伟才,席炎炎,肖红,等. 燃料组件导向管冷壁效应对CHF影响试验研究[J]. 原子能科学技术,2022, 56(6): 1212-1218. doi: 10.7538/yzk.2021.youxian.0925 [14] OWEN D B. Factors for one-sided tolerance limits and for variables sampling plans: SCR-607[R]. Albuquerque: Sandia National Lab. , 1963. [15] LAHEY JR R T, SHIRALKAR B S, RADCLIFFE D W. Two-phase flow and heat transfer in multirod geometries: subchannel and pressure drop measurements in a nine-rod bundle for diabatic and adiabatic conditions: NSA-24-031579[R]. San Jose: General Electric Co. , 1970. [16] BLYTH T, AVRAMOVA M. Development and implementation of CFD-informed models for the advanced subchannel code CTF: ORNL/TM-2017/191[R]. Oak Ridge: Oak Ridge National Laboratory, 2017. -
计量
- 文章访问数: 52
- HTML全文浏览量: 18
- PDF下载量: 19
- 被引次数: 0
