Modified SST k-ω-γ Model and Prediction of Laminar to Turbulent Flow Transition in Helical Tube
-
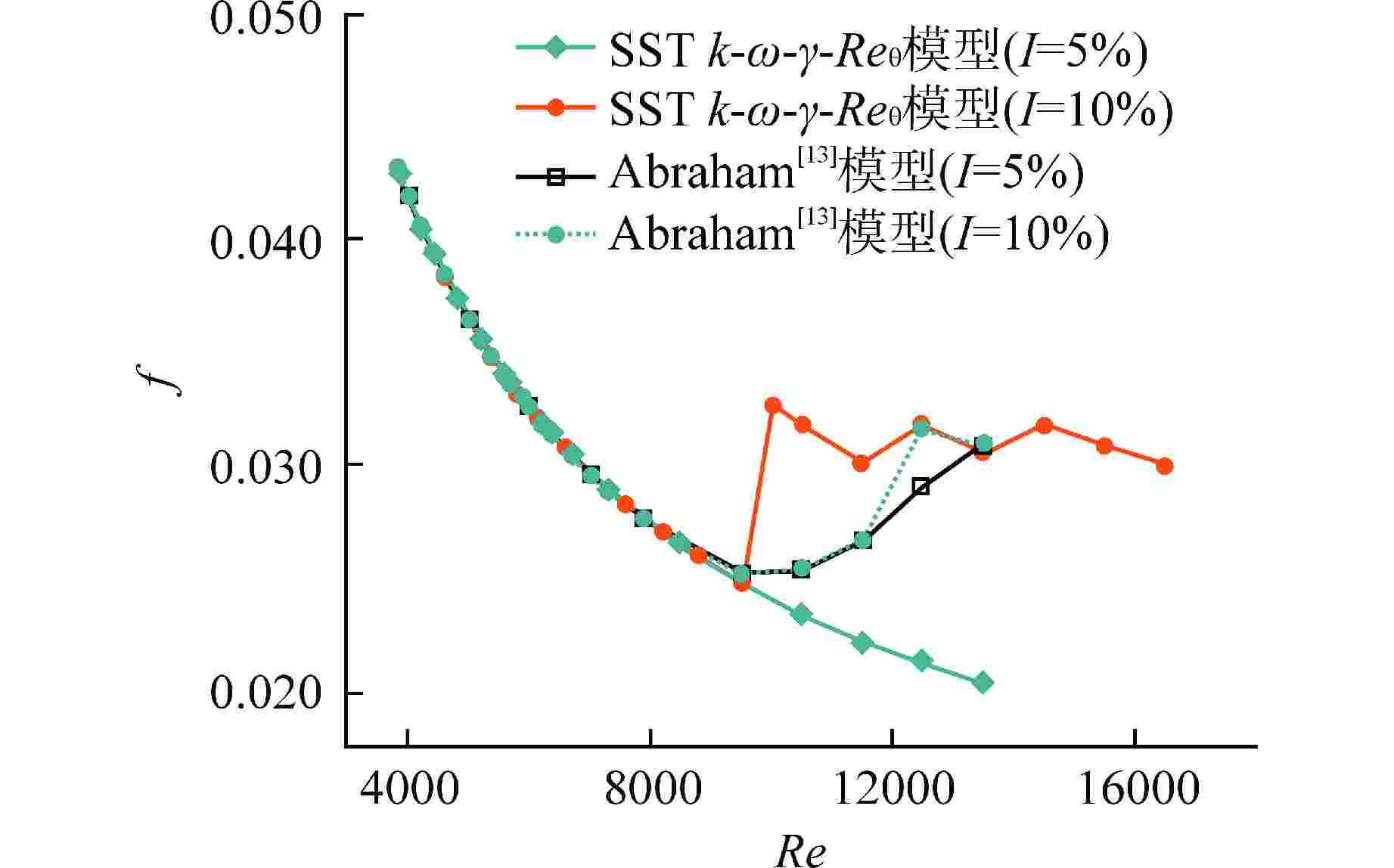
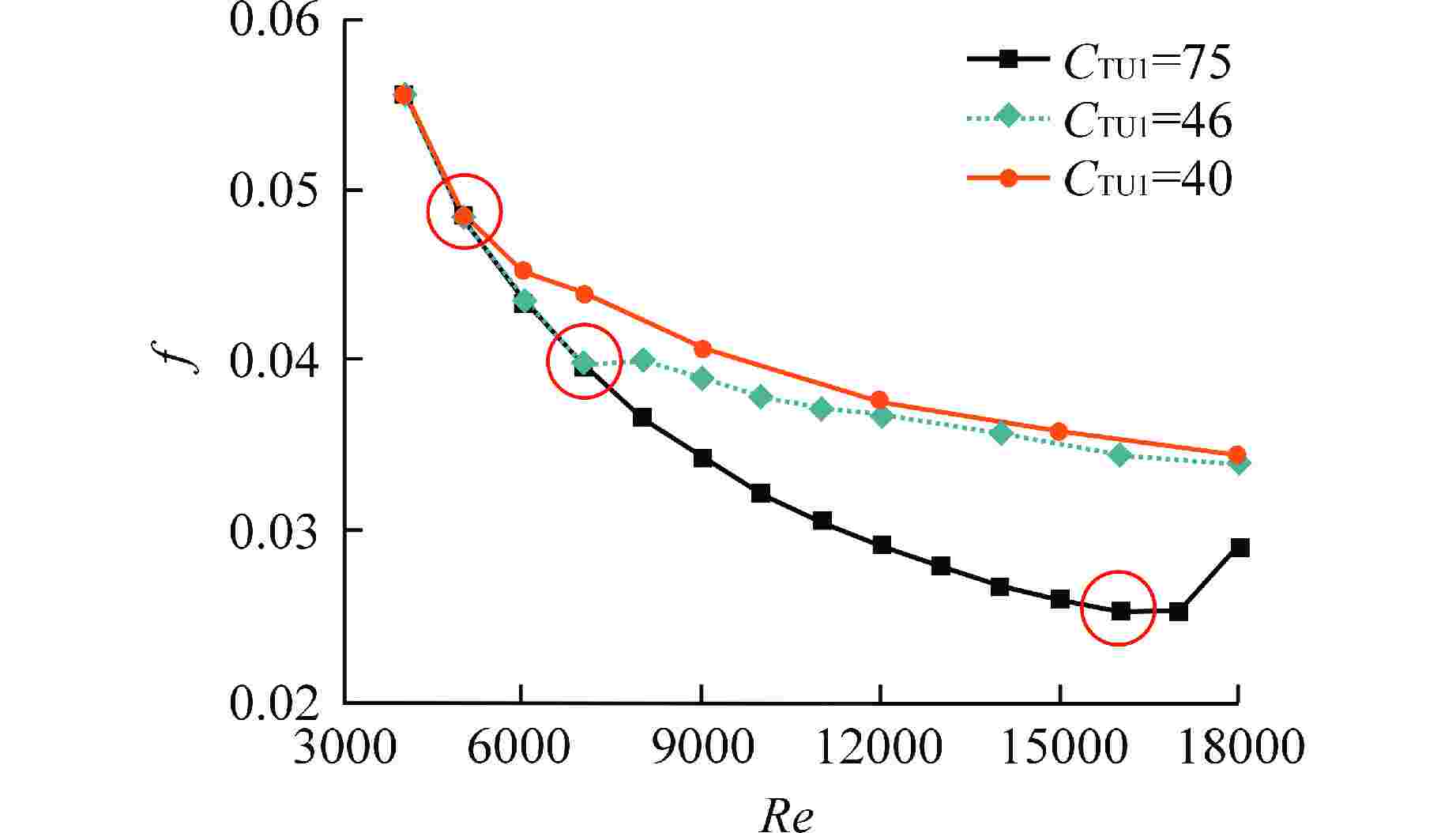
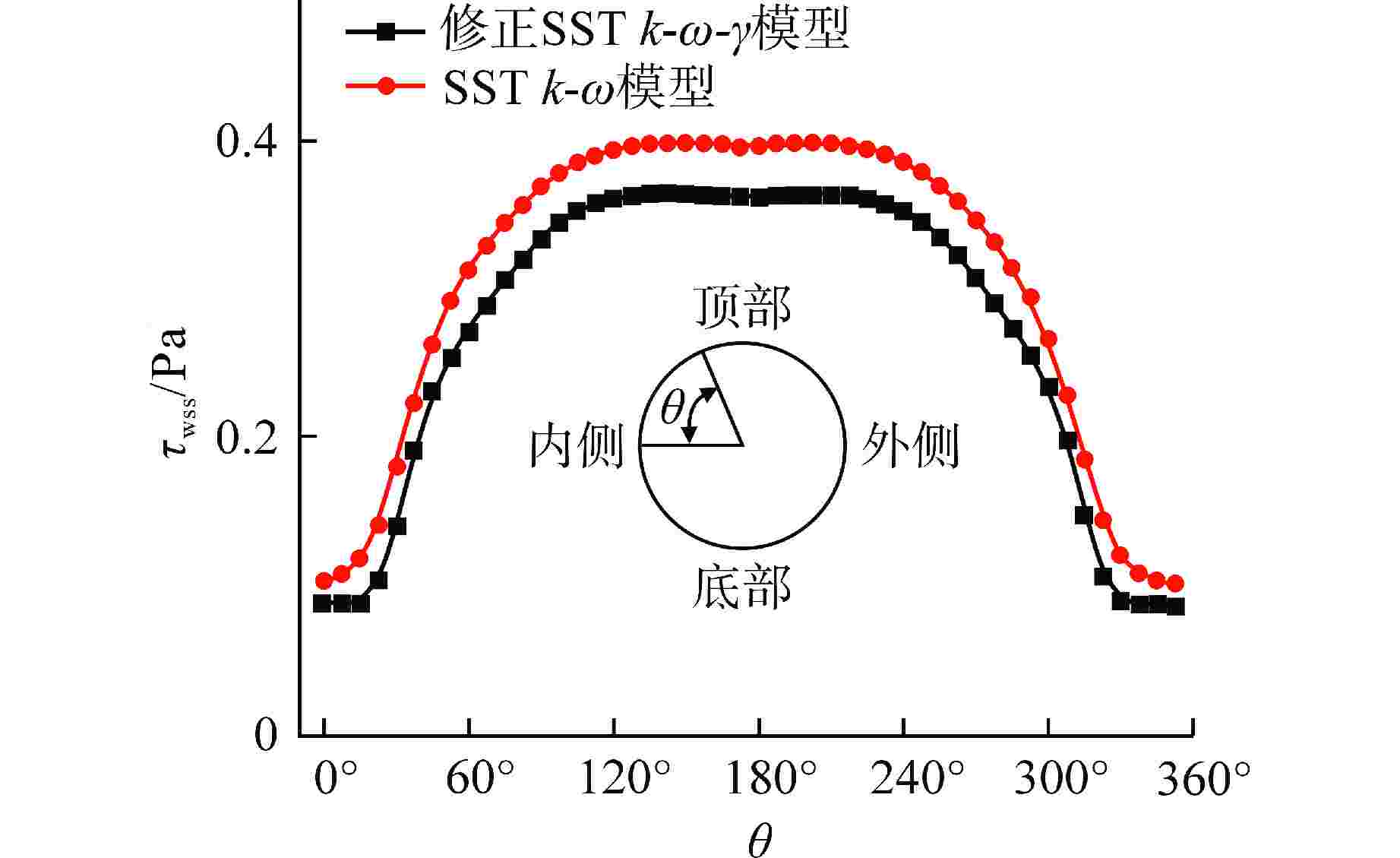
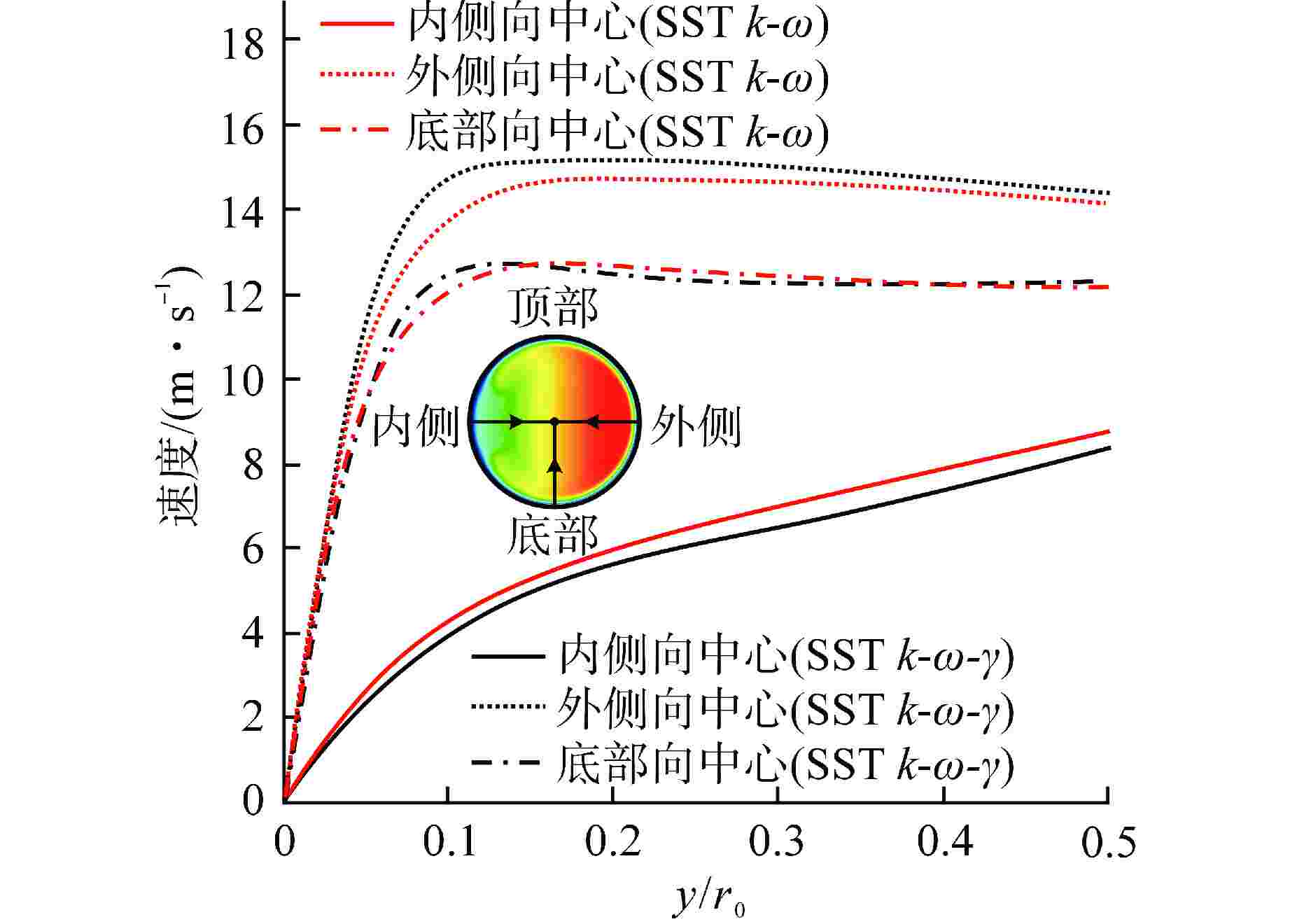
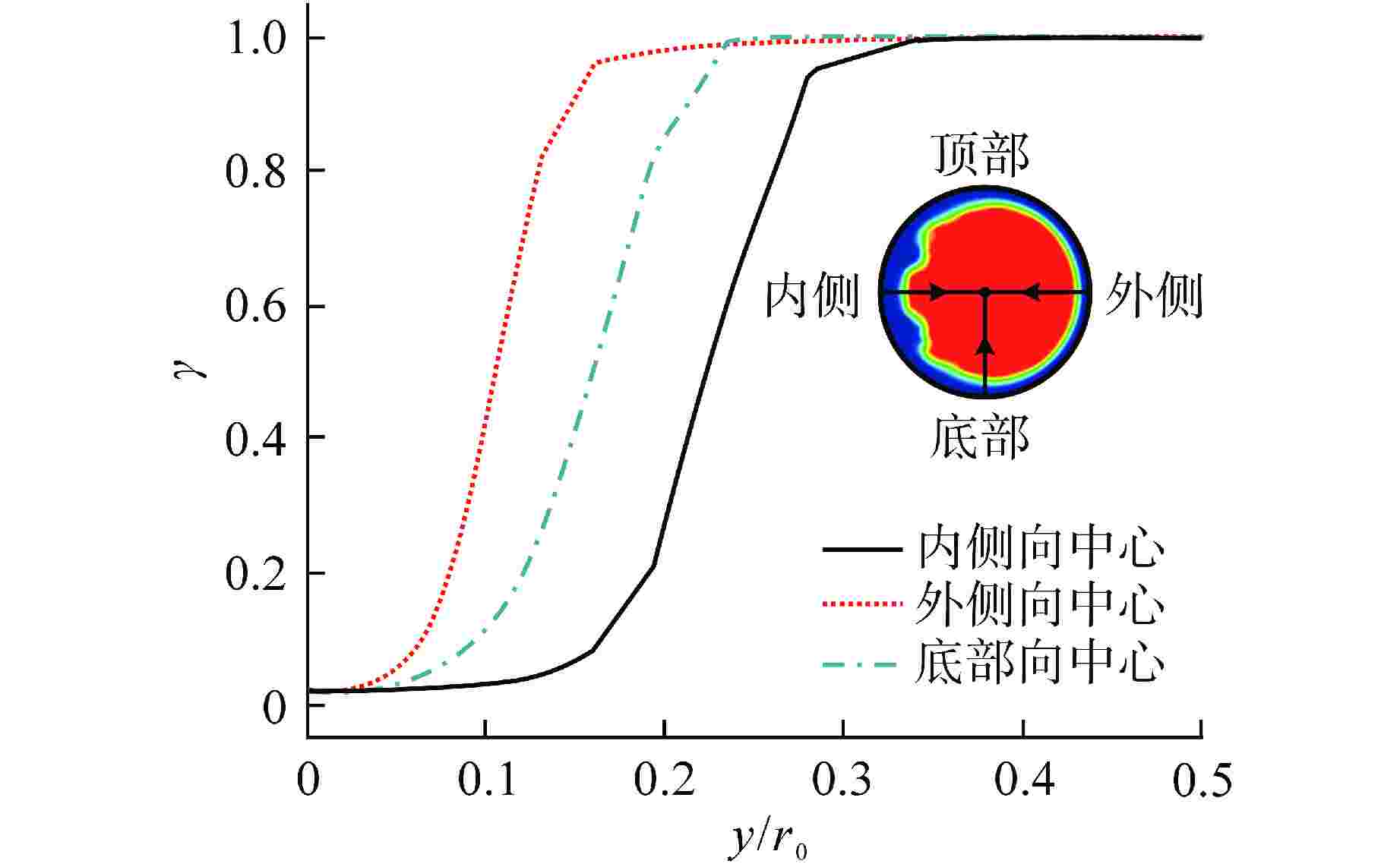
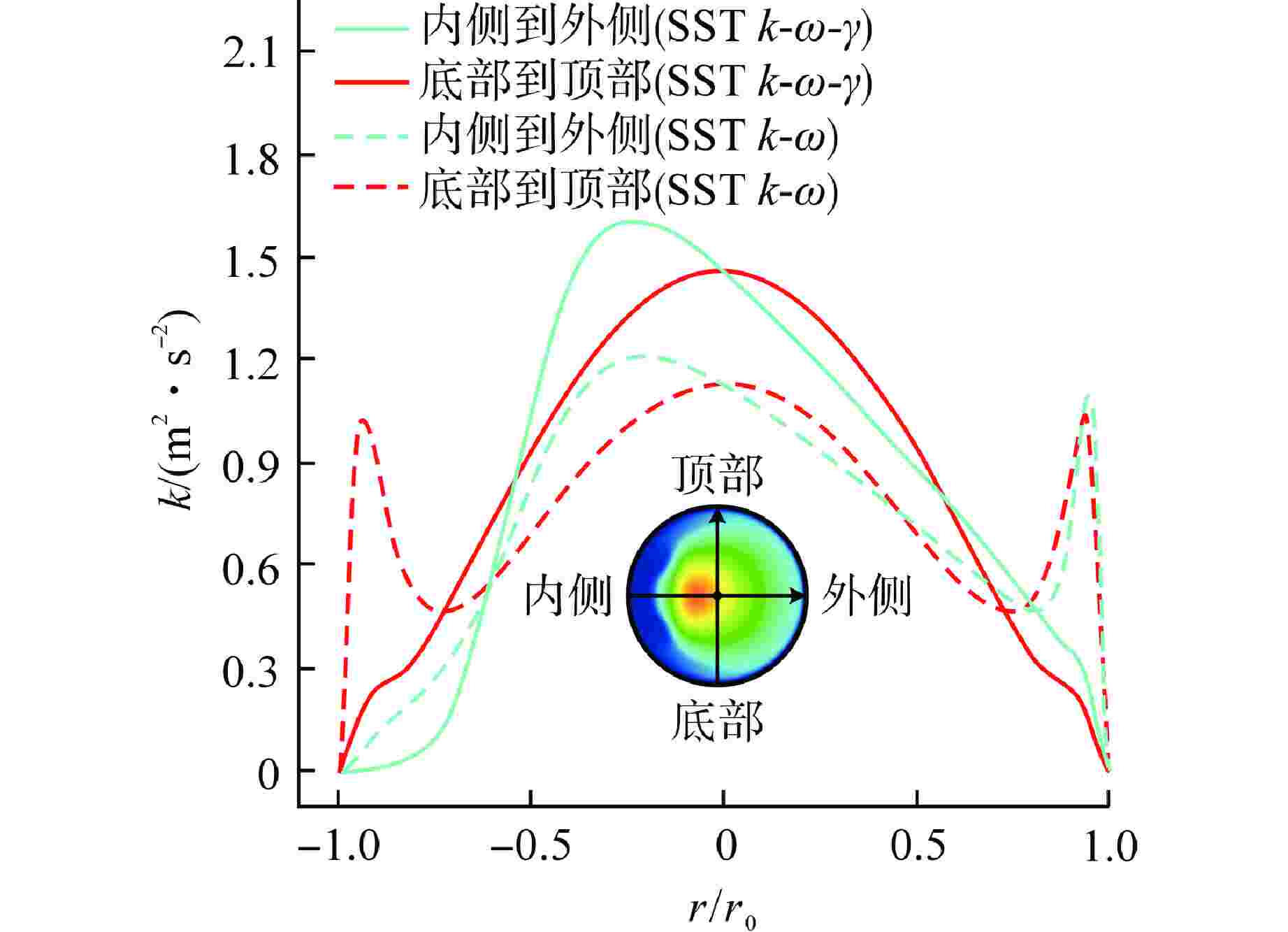
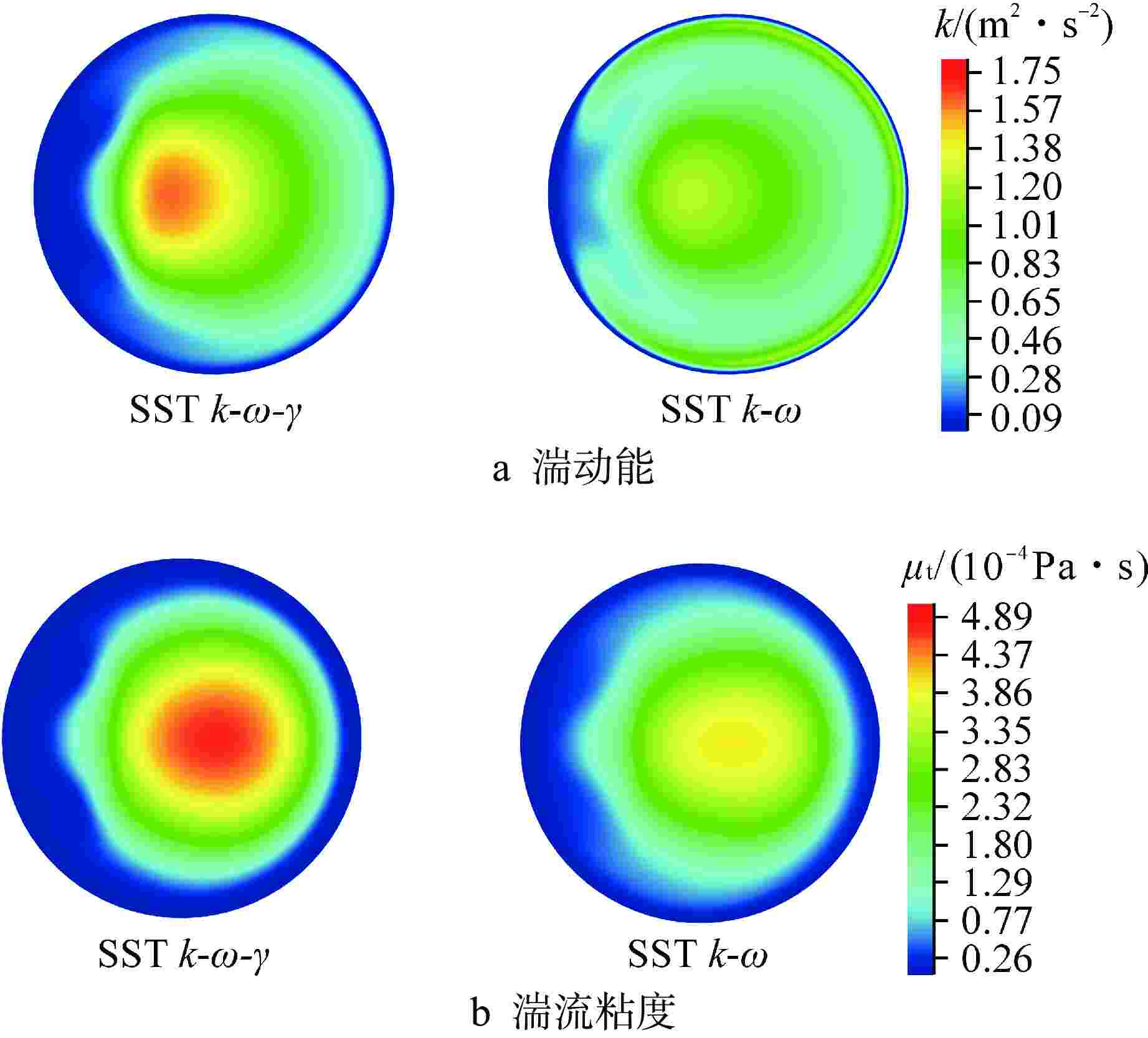
摘要: 螺旋管式换热器因具有结构紧凑和换热性能好等优点,在各领域被广泛应用。区别于直管内流动,流体在螺旋管内流动时会受到离心力作用。由于离心力的存在,螺旋管内层流向湍流转捩的临界雷诺数会随着螺旋曲率的增大而增大。本文采用数值方法研究了螺旋管内层流向湍流的转捩过程,通过分析阻力系数随雷诺数的变化关系,对比剪切应力输运(SST) k-ω-γ-Reθ模型和SST k-ω-γ模型模拟转捩过程的准确性,分析了不同入口湍流强度(5%、10%)对计算结果的影响。SST k-ω-γ-Reθ模型对入口湍流强度较敏感,而SST k-ω-γ模型受入口湍流强度影响较小。与前人经验关系式相比,SST k-ω-γ模型模拟得到的临界雷诺数偏大。本文通过调节SST k-ω-γ模型中γ输运方程的经验系数,发现经验系数CTU1对转捩起始点有较大影响,同一曲率下,临界雷诺数随CTU1增大而增大。本文基于现有经验公式确定了不同曲率螺旋管(0.02、0.04、0.06、0.11)所对应的CTU1,并拟合得到螺旋曲率和CTU1的关联式,验证了修正后SST k-ω-γ模型模拟螺旋管内层流和湍流阻力系数的准确性,比较了SST k-ω模型和修正SST k-ω-γ模型计算结果中速度、湍动能以及湍流粘度等变量的不同。Abstract: Helical tube heat exchangers are widely used in various fields due to compactness and good heat transfer performance. Different from the flow in a straight pipe, the fluid will be subjected to centrifugal force when flowing in a helical tube. Due to the existence of centrifugal force, the critical Reynolds number for the transition from laminar to turbulent flow in the helical tube increases with the increasing of helical curvature ratios. In the current investigation, the transition process from laminar to turbulent flow in the helical tube is studied by numerical method. By analyzing the relationship between the resistance coefficient and Reynolds number, the accuracy of simulating the transition process by the shear stress transport (SST) k-ω-γ-Reθ model and SST k-ω-γ model is compared, and the effects of different inlet turbulence intensities (5% and 10%) on the calculation results are analyzed. The SST k-ω-γ-Reθ model is more sensitive to inlet turbulent intensities, while the SST k-ω-γ model is less affected by inlet turbulent intensities. The critical Reynolds number obtained by SST k-ω-γ model is larger than the critical Reynolds number calculated by the empirical correlation in the literature. In this paper, by adjusting the empirical coefficient of γ transport equation in SST k-ω-γ model, it is found that CTU1 has a significant influence on the onset of the transition process. With the same helical curvature ratio, the critical Reynolds number increases with the increasing of CTU1. In the current investigation, based on the existing empirical formula, the CTU1 for different helical curvature ratios (δ=0.02, 0.04, 0.06, and 0.11) are determined, and the correlation between helical curvature and CTU1 is obtained by fitting. The accuracy of the modified SST k-ω-γ model in simulating the laminar and turbulent friction factors in helical tubes is verified, and the differences of velocity, turbulent kinetic energy and turbulent viscosity in the calculation results of SST k-ω model and modified SST k-ω-γ model are compared.
-
Key words:
- Helical tube /
- Friction factor /
- Laminar flow /
- Turbulent flow /
- Transition
-
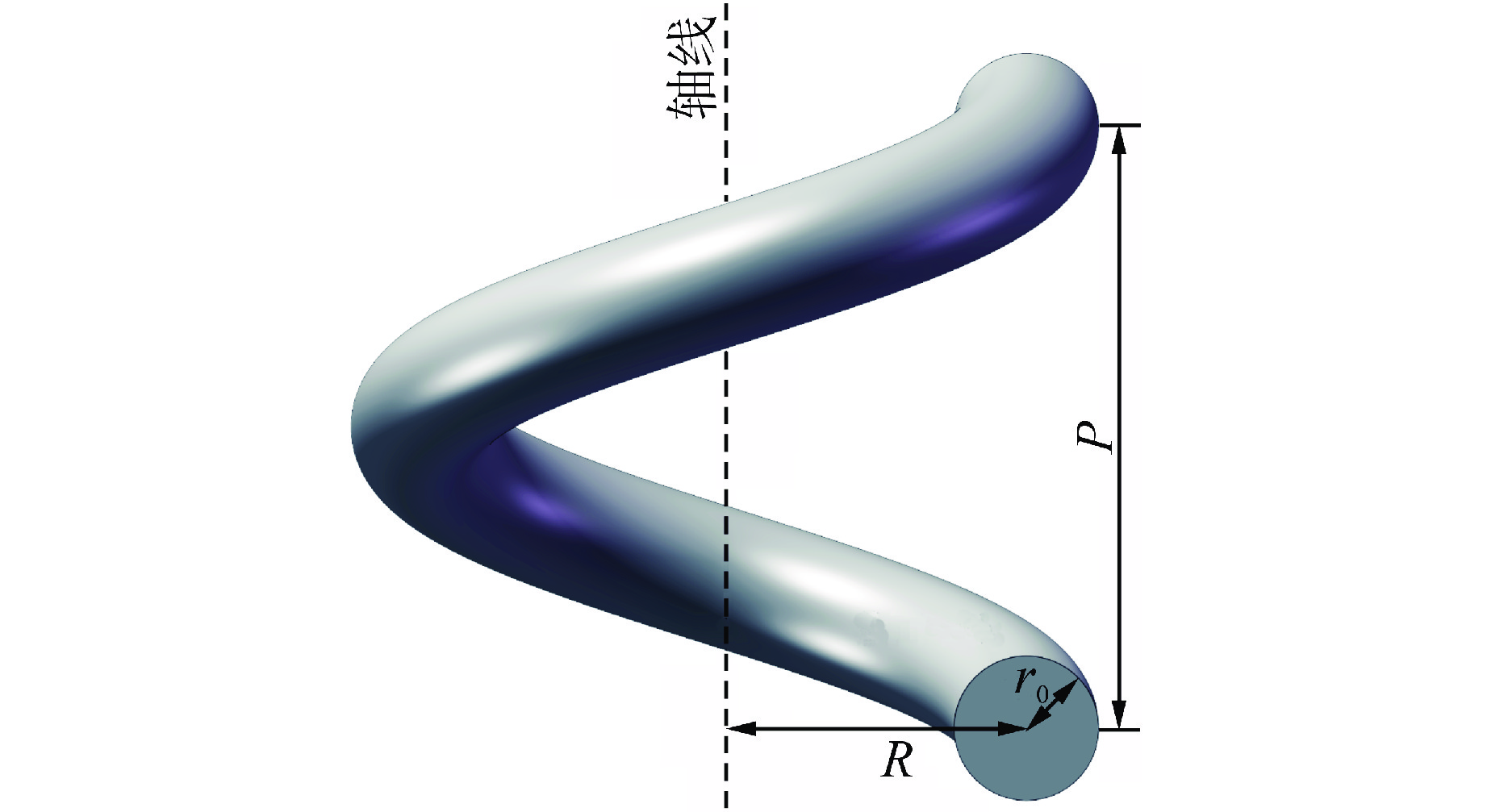
表 1 不同曲率螺旋管几何参数
Table 1. Geometric Parameters of Helical Tubes with Different Curvatures
δ δmod r0/mm R/mm P/mm 0.02 0.0195 4 200 31 0.04 0.0390 4 100 16.5 0.06 0.0584 6 100 17 0.11 0.1038 4 36 16 表 2 不同网格间的最大相对误差
Table 2. Maximum Relative Error between Different Grids
δ 低精度网格数量/105 高精度网格数量/105 ζmax/% 0.02 33.6 80.9 0.96 0.04 25.0 55.6 1.00 0.06 23.6 75.2 0.78 表 3 不同模型经验系数所计算的阻力系数
Table 3. Friction Factors with Different Model Coefficients
经验系数CTU1 经验系数CTU3 经验系数β* f 60 1 0.09 0.0334 70 1 0.09 0.0293 80 1 0.09 0.0248 90 1 0.09 0.0248 100 1.1 0.09 0.0248 100 1.2 0.09 0.0248 100 1.3 0.09 0.0248 100 1.4 0.09 0.0248 100 1 0.095 0.0247 100 1 0.098 0.0247 100 1 0.101 0.0247 100 1 0.104 0.0246 表 4 不同曲率下的CTU1值
Table 4. CTU1 for Different δmod
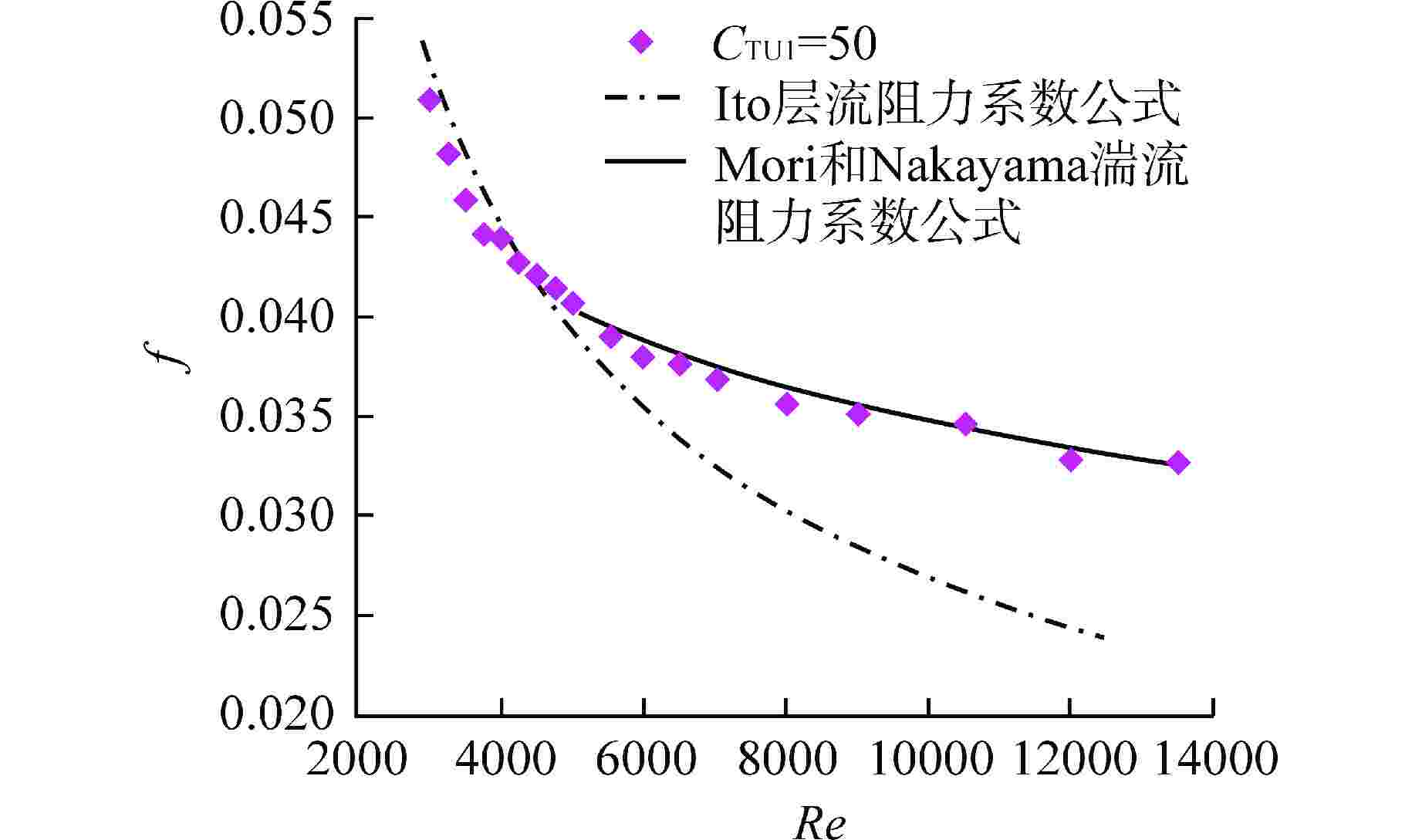
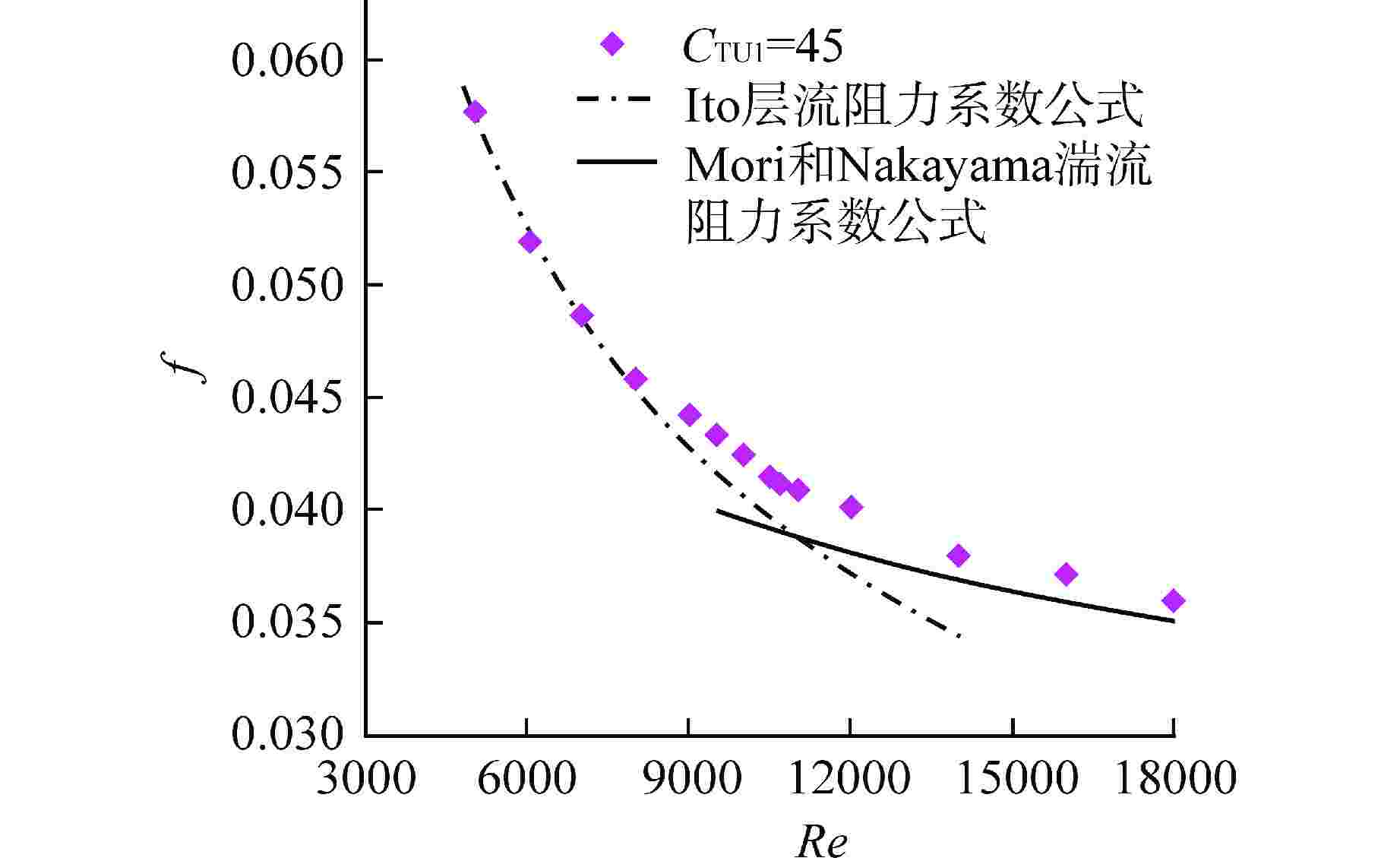
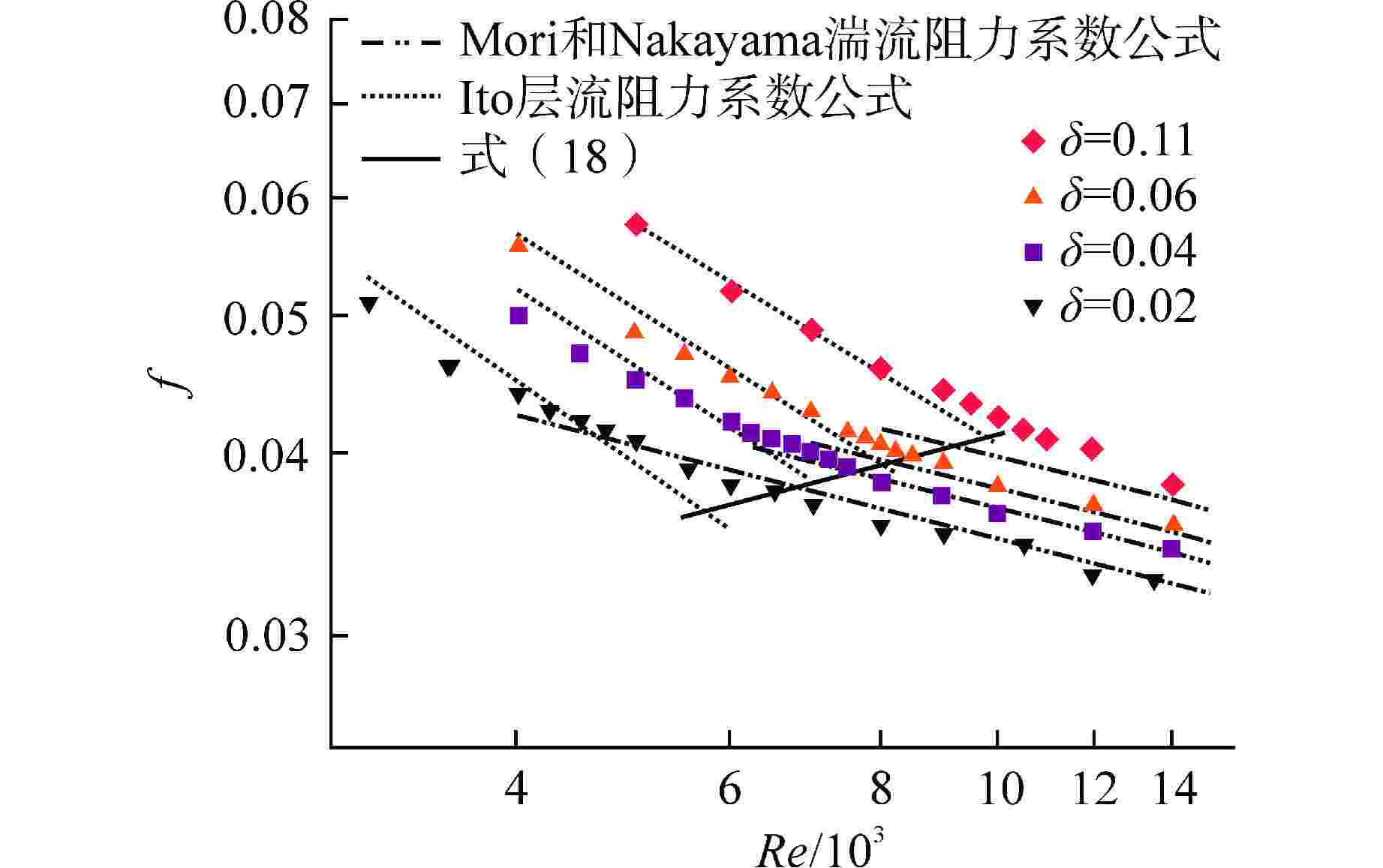
δmod 推荐CTU1 Recri 数值结果 式(18) 0.0195 50 5600 5700 0.0390 47 7300 7100 0.0584 46 8500 8100 0.1038 45 10700 9700 -
[1] ZHAO H J, LI X W, WU X X. New friction factor equations developed for turbulent flows in rough helical tubes[J]. International Journal of Heat and Mass Transfer, 2016, 95: 525-534. doi: 10.1016/j.ijheatmasstransfer.2015.12.035 [2] ZHAO H J, LI X W, WU X X. New friction factor and Nusselt number equations for turbulent convection of liquids with variable properties in circular tubes[J]. International Journal of Heat and Mass Transfer, 2018, 124: 454-462. doi: 10.1016/j.ijheatmasstransfer.2018.03.082 [3] 李晓伟,吴莘馨,张作义,等. 高温气冷堆示范工程螺旋管式直流蒸汽发生器工程验证试验[J]. 清华大学学报: 自然科学版,2021, 61(4): 329-337. [4] LI X W, GAO W K, SU Y, et al. Thermal analysis of HTGR helical tube once through steam generators using 1D and 2D methods[J]. Nuclear Engineering and Design, 2019, 355: 110352. doi: 10.1016/j.nucengdes.2019.110352 [5] LANGTRY R B, MENTER F R. Correlation-based transition modeling for unstructured parallelized computational fluid dynamics codes[J]. AIAA Journal, 2009, 47(2): 2894-2906. [6] CONTENT C, HOUDEVILLE R. Application of the γ-Rθ laminar-turbulent transition model in Navier-Stokes computations[C]//40th Fluid Dynamics Conference and Exhibit. Chicago: AIAA, 2010. [7] MALAN P, SULUKSNA K, JUNTASARO E. Calibrating the γ-Reθ transition model for commercial CFD[C]//47th AIAA Aerospace Sciences Meeting including The New Horizons Forum and Aerospace Exposition. Orlando: AIAA, 2009. [8] GRABE C, KRUMBEIN A. Extension of the γ-Reθt model for prediction of crossflow transition[C]//52nd Aerospace Sciences Meeting. National Harbor: AIAA, 2014. [9] MEDIDA S, BAEDER J. A new crossflow transition onset criterion for RANS turbulence models[C]//21st AIAA Computational Fluid Dynamics Conference. San Diego: AIAA, 2013. [10] DASSLER P, KOZULOVIC D, FIALA A. Transport equation for roughness effects on laminar-turbulent transition[C]//The 15th International Conference on Fluid Flow Technologies. Budapest, Hungary: CMFF, 2012. [11] MENTER F R, SMIRNOV P E, LIU T, et al. A one-equation local correlation-based transition model[J]. Flow, Turbulence and Combustion, 2015, 95(4): 583-619. doi: 10.1007/s10494-015-9622-4 [12] ABRAHAM J P, SPARROW E M, GORMAN J M, et al. Application of an intermittency model for laminar, transitional, and turbulent internal flows[J]. Journal of Fluids Engineering, 2019, 141(7): 071204. doi: 10.1115/1.4042664 [13] DI PIAZZA I, CIOFALO M. Transition to turbulence in toroidal pipes[J]. Journal of Fluid Mechanics, 2011, 687: 72-117. doi: 10.1017/jfm.2011.321 [14] ZHAO H J, LI X W, WU Y J, et al. Friction factor and Nusselt number correlations for forced convection in helical tubes[J]. International Journal of Heat and Mass Transfer, 2020, 155: 119759. doi: 10.1016/j.ijheatmasstransfer.2020.119759 [15] ITO H. Theoretical and experimental investigation concerning the flow through curved pipes[C]//Mem Inst High Speed Mech. Tohoku University, 1959. [16] MORI Y, NAKAYAMA W. Study of forced convective heat transfer in curved pipes (2nd report, turbulent region)[J]. International Journal of Heat and Mass Transfer, 1967, 10(1): 37-59. -





 下载:
下载: